12 In Simplest Radical Form

Quick Links :

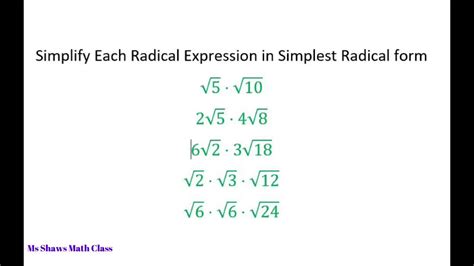
The world of radicals and algebra can be a daunting one, especially when it comes to simplifying expressions. However, with the right approach and techniques, anyone can master the art of simplifying radicals. In this article, we will delve into the world of radicals, explore the concept of simplest radical form, and provide practical examples to help solidify understanding.

What are Radicals?

Radicals are mathematical expressions that contain a square root or other root symbols. They are used to represent numbers that cannot be expressed as a finite decimal or fraction. Radicals are commonly used in algebra, geometry, and calculus to solve equations and manipulate expressions.

Why Simplify Radicals?

Simplifying radicals is an essential skill in mathematics, as it allows us to:

• Reduce complex expressions to a more manageable form
• Eliminate unnecessary roots and simplify calculations
• Identify equivalent expressions and make algebraic manipulations easier
• Improve problem-solving efficiency and accuracy

Simplifying Radicals: The Basics

To simplify radicals, we need to follow a set of rules and guidelines. Here are the basic steps:

1. Factor the radicand: The radicand is the number inside the radical symbol. Factor the radicand into its prime factors to identify any perfect squares or cubes.
2. Identify perfect squares or cubes: Look for any perfect squares or cubes among the prime factors. These can be simplified using the properties of radicals.
3. Simplify the radical: Use the properties of radicals to simplify the expression. For example, √(x^2) = x, and ³√(x^3) = x.

Properties of Radicals

Here are some key properties of radicals that will help you simplify expressions:

• Product property: √(ab) = √a × √b
• Quotient property: √(a/b) = √a / √b
• Power property: √(a^m) = a^(m/2)

Examples of Simplifying Radicals

Let's practice simplifying radicals with some examples:

• √(16) = √(4^2) = 4
• √(8) = √(4 × 2) = √4 × √2 = 2√2
• ³√(27) = ³√(3^3) = 3

Real-World Applications of Simplifying Radicals

Simplifying radicals has numerous real-world applications, including:

• Physics and engineering: Radicals are used to describe the motion of objects, forces, and energies.
• Computer science: Radicals are used in algorithms for solving complex equations and optimizing functions.
• Data analysis: Radicals are used in statistical models to analyze and visualize data.

Common Mistakes When Simplifying Radicals

When simplifying radicals, it's easy to make mistakes. Here are some common errors to watch out for:

• Forgetting to factor the radicand: Always factor the radicand before simplifying the radical.
• Not identifying perfect squares or cubes: Take the time to identify any perfect squares or cubes among the prime factors.
• Misapplying properties of radicals: Make sure to apply the properties of radicals correctly to avoid errors.

Best Practices for Simplifying Radicals

To become proficient in simplifying radicals, follow these best practices:

• Practice regularly: Regular practice will help you develop muscle memory and improve your skills.
• Use online resources: Utilize online resources, such as video tutorials and practice problems, to supplement your learning.
• Break down complex expressions: Break down complex expressions into simpler components to make simplification easier.

Conclusion

Simplifying radicals is an essential skill in mathematics that requires practice, patience, and attention to detail. By following the rules and guidelines outlined in this article, you'll become proficient in simplifying radicals and be able to tackle complex algebraic expressions with confidence.

We hope this article has been informative and helpful. If you have any questions or comments, please don't hesitate to reach out. Share this article with your friends and colleagues who may benefit from learning about simplifying radicals.