Simplify 105 In Simplest Radical Form

Quick Links :

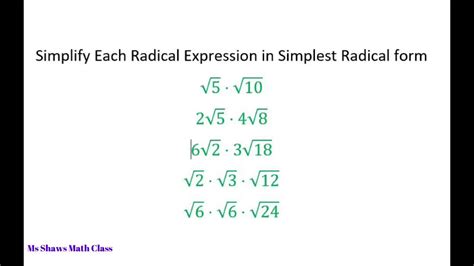
The task of simplifying √105 involves breaking down the number 105 into its prime factors and then simplifying the radical expression.

Understanding the Basics of Simplifying Radicals

Radicals are simplified by finding the prime factors of the number inside the square root. For example, √12 can be simplified by breaking down 12 into its prime factors, which are 2, 2, and 3. This gives us √(2^2 * 3), which simplifies to 2√3.

Prime Factorization of 105

To simplify √105, we first need to find the prime factors of 105. The prime factorization of 105 is 3, 5, and 7, since 3 * 5 * 7 = 105.

Breaking Down 105 into Prime Factors

• 105 = 3 * 5 * 7
• √105 = √(3 * 5 * 7)

Simplifying the Radical Expression

Since we cannot simplify the radical expression √(3 * 5 * 7) further, we leave it as it is. However, we can rewrite it as:

√105 = √(3 * 5 * 7) = √(3 * 5) * √7 = √15 * √7

However, it's worth noting that √105 is often left in its original form rather than being broken down into simpler radicals.

Using this Simplification in Math Problems

When dealing with math problems that involve radicals, it's essential to simplify the radical expressions to their simplest forms. This helps in making calculations easier and more manageable.

Example Problem

Find the value of √105 + √15:

Since we've already simplified √105, we can now use it to solve this problem.

√105 + √15 = √15 * √7 + √15 = √15(√7 + 1)

This is the simplified form of the expression.

Best Practices for Simplifying Radicals

When simplifying radicals, it's crucial to:

• Find the prime factors of the number inside the square root.
• Break down the number into its simplest radical form.
• Use parentheses to group the prime factors correctly.
• Simplify the radical expression as much as possible.

Common Mistakes to Avoid

• Not finding the prime factors correctly.
• Not simplifying the radical expression fully.
• Not using parentheses correctly.

Conclusion

Simplifying radicals is an essential skill in mathematics, and it's used in various math problems and equations. By following the steps outlined above and using the best practices, you can simplify radicals like a pro.

Call to Action

Practice simplifying radicals regularly to improve your math skills. Try solving different math problems that involve radicals and simplify the expressions to their simplest forms.

Share your thoughts and experiences with simplifying radicals in the comments below. Do you have any tips or tricks for simplifying radicals? Let us know!