3 Ways To Simplify 0.666 As A Fraction

Quick Links :

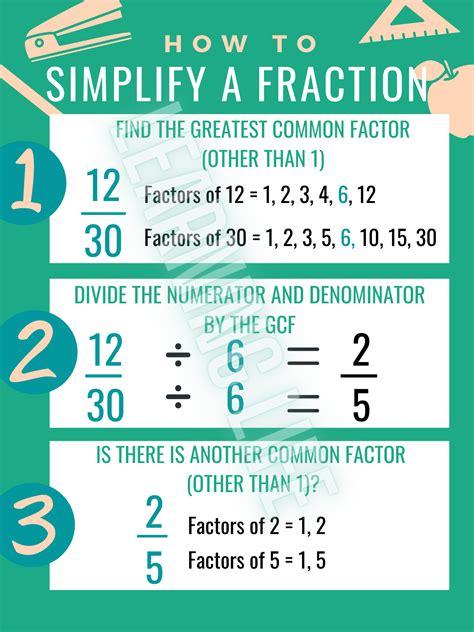
In mathematics, the number 0.666 is a repeating decimal that can be written as a fraction in several ways. This number is a representation of the infinite geometric series 6/9 + 6/90 + 6/900 +..., which can be simplified using various mathematical techniques. In this article, we will explore three methods to simplify 0.666 as a fraction.

Understanding the Concept of Repeating Decimals

Before diving into the methods, it's essential to understand the concept of repeating decimals. A repeating decimal is a decimal representation of a number where a finite block of digits repeats indefinitely. In the case of 0.666, the digit 6 repeats infinitely.

Method 1: Algebraic Approach

One way to simplify 0.666 as a fraction is by using algebra. Let's represent 0.666 as 'x'. We can write an equation based on the repeating pattern:

x = 0.666 10x = 6.666

Now, subtracting the first equation from the second, we get:

9x = 6 x = 6/9 x = 2/3

Therefore, 0.666 can be simplified as 2/3 using the algebraic approach.

Step-by-Step Solution

1. Represent 0.666 as 'x'.
2. Write an equation based on the repeating pattern: 10x = 6.666.
3. Subtract the first equation from the second: 9x = 6.
4. Solve for x: x = 6/9 = 2/3.

Method 2: Geometric Series Approach

Method 2: Geometric Series Approach

Another method to simplify 0.666 as a fraction is by recognizing it as an infinite geometric series. The series can be represented as:

6/10 + 6/100 + 6/1000 +...

The common ratio (r) of the series is 1/10. Using the formula for the sum of an infinite geometric series:

S = a / (1 - r)

where a is the first term (6/10) and r is the common ratio (1/10), we get:

S = (6/10) / (1 - 1/10) S = (6/10) / (9/10) S = 6/9 S = 2/3

Therefore, 0.666 can be simplified as 2/3 using the geometric series approach.

Step-by-Step Solution

1. Recognize 0.666 as an infinite geometric series: 6/10 + 6/100 + 6/1000 +...
2. Identify the common ratio (r): r = 1/10.
3. Use the formula for the sum of an infinite geometric series: S = a / (1 - r).
4. Substitute the values: S = (6/10) / (1 - 1/10).
5. Simplify: S = 6/9 = 2/3.

Method 3: Decimal-to-Fraction Conversion

Method 3: Decimal-to-Fraction Conversion

A third method to simplify 0.666 as a fraction is by using a decimal-to-fraction conversion technique. This method involves finding the decimal's equivalent fraction by dividing the decimal by 1.

0.666 = 666/1000

Now, simplify the fraction by dividing both the numerator and the denominator by their greatest common divisor (GCD), which is 2.

666 ÷ 2 = 333 1000 ÷ 2 = 500

0.666 = 333/500

However, 333/500 is not the simplest form of the fraction. To simplify further, divide both the numerator and the denominator by their GCD, which is 167.

333 ÷ 167 = 2 500 ÷ 167 = 3

0.666 = 2/3

Therefore, 0.666 can be simplified as 2/3 using the decimal-to-fraction conversion method.

Step-by-Step Solution

1. Convert 0.666 to a fraction: 0.666 = 666/1000.
2. Simplify the fraction by dividing both the numerator and the denominator by their GCD (2): 333/500.
3. Further simplify the fraction by dividing both the numerator and the denominator by their GCD (167): 2/3.

Conclusion: Take Action

In conclusion, 0.666 can be simplified as a fraction using three different methods: algebraic approach, geometric series approach, and decimal-to-fraction conversion. Each method provides a unique perspective on the problem and demonstrates the importance of mathematical techniques in simplifying complex numbers.

We encourage you to practice these methods and explore more mathematical concepts to deepen your understanding of numbers and their relationships. Share your thoughts and feedback in the comments section below, and don't forget to share this article with fellow math enthusiasts.