Simplify 0.4 Fraction To Lowest Terms

Quick Links :

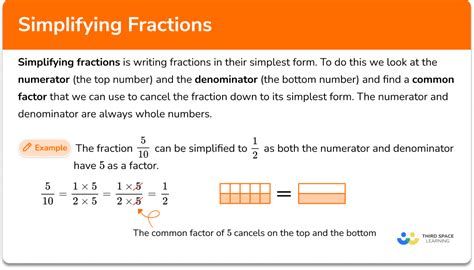
Simplifying a fraction involves finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by this GCD. The fraction 0.4 is not in fractional form, so first, we need to convert it into a fraction.

0.4 is equal to 4/10. To simplify this fraction, we find the GCD of 4 and 10, which is 2. Then, we divide both the numerator and denominator by this GCD.

So, 4/10 becomes (4/2)/(10/2) = 2/5.

Therefore, 0.4 simplified to its lowest terms is 2/5.

Understanding Fractions

A fraction is a way to express a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number), separated by a line. The numerator tells us how many equal parts we have, and the denominator tells us how many parts the whole is divided into.

For example, in the fraction 2/5, the numerator is 2, and the denominator is 5. This fraction represents 2 out of 5 equal parts.

Importance of Simplifying Fractions

Simplifying fractions is crucial for several reasons:

1. Easier Comparison: Simplified fractions make it easier to compare different fractions. For instance, 1/2 and 2/4 are the same, but in their simplified form, 1/2 is more straightforward.

2. Simpler Calculations: Working with simplified fractions often simplifies calculations. When adding, subtracting, multiplying, or dividing fractions, having them in their simplest form reduces the complexity of the operations.

3. Clearer Representation: Simplified fractions provide a clearer representation of the proportion or part of the whole they represent. This clarity is essential in mathematics and real-world applications where precision and understanding are critical.

Steps to Simplify a Fraction

1. Identify the Fraction: Start by identifying the fraction you want to simplify. It should be in the form of a/b, where 'a' is the numerator and 'b' is the denominator.

2. Find the GCD: Determine the greatest common divisor (GCD) of the numerator and denominator. The GCD is the largest number that divides both the numerator and denominator without leaving a remainder.

3. Divide by the GCD: Once you have the GCD, divide both the numerator and the denominator by this number. The result will be the simplified fraction.

Practical Applications of Fractions

Fractions are not just mathematical concepts; they have numerous practical applications in everyday life and various professions. Here are a few examples:

1. Cooking and Recipes: Fractions are essential in cooking, especially when dealing with ingredient measurements. Recipes often call for fractions of a cup, teaspoon, or other units of measurement.

2. Building and Construction: Fractions are used extensively in building and construction to measure lengths, widths, and heights. Precision is crucial in these fields, and fractions provide the necessary accuracy.

3. Science and Engineering: In science and engineering, fractions are used to express proportions, concentrations, and ratios. They are fundamental in understanding and describing physical phenomena.

Conclusion

Simplifying fractions is a fundamental skill in mathematics and has significant practical applications. Understanding how to simplify fractions to their lowest terms enhances mathematical literacy and problem-solving abilities. The process involves identifying the fraction, finding the greatest common divisor of the numerator and denominator, and then dividing both by this GCD. By mastering this skill, individuals can better grasp mathematical concepts and apply them effectively in real-world scenarios.

We hope this explanation has helped you understand how to simplify fractions. If you have any specific questions or need further clarification, please don't hesitate to ask.

Share Your Thoughts

We invite you to share your thoughts on simplifying fractions or ask any questions you might have in the comments section below. Your input is valuable, and we look forward to engaging with you.