Understanding the standard form of a linear equation is crucial in mathematics, particularly in algebra. The standard form of a linear equation is typically represented as Ax + By = C, where A, B, and C are constants. In this article, we'll delve into the process of converting a linear equation from slope-intercept form (Y = mx + b) to standard form.
Why Convert to Standard Form?

Converting a linear equation to standard form is essential in various mathematical operations, such as graphing, finding the x-intercept, and solving systems of equations. The standard form provides a clear and concise way to represent linear equations, making it easier to perform these operations.
Understanding the Slope-Intercept Form

The slope-intercept form of a linear equation is represented as Y = mx + b, where m is the slope and b is the y-intercept. The slope (m) represents the rate of change of the line, while the y-intercept (b) is the point where the line crosses the y-axis.
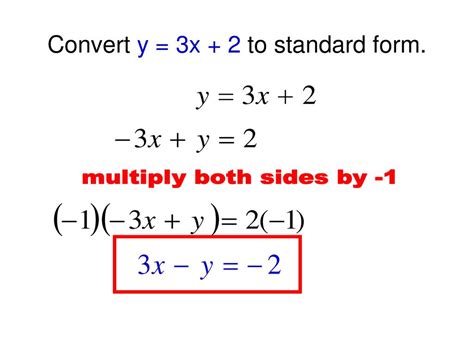
Converting Y = 3x + 4 to Standard Form
To convert the equation Y = 3x + 4 to standard form, we need to rearrange the terms to isolate the variables (x and y) on one side of the equation and the constants on the other side. We can achieve this by subtracting 3x from both sides of the equation and then multiplying both sides by -1.
Y = 3x + 4 Y - 3x = 4 -3x + Y = 4 -3x - Y = -4
The resulting equation is -3x - Y = -4, which is in standard form.
Key Takeaways for Converting to Standard Form

When converting a linear equation from slope-intercept form to standard form, remember to:
- Isolate the variables (x and y) on one side of the equation.
- Move the constants to the other side of the equation.
- Rearrange the terms to achieve the standard form Ax + By = C.
By following these steps, you can easily convert any linear equation from slope-intercept form to standard form.
Practical Applications of Standard Form

The standard form of a linear equation has numerous practical applications in various fields, including:
- Graphing: Standard form makes it easier to graph linear equations on a coordinate plane.
- Finding the x-intercept: Standard form allows you to find the x-intercept by setting y = 0 and solving for x.
- Solving systems of equations: Standard form is essential for solving systems of linear equations using methods like substitution and elimination.
Common Mistakes to Avoid When Converting to Standard Form

When converting a linear equation to standard form, be aware of the following common mistakes:
- Forgetting to isolate the variables on one side of the equation.
- Failing to move the constants to the other side of the equation.
- Not rearranging the terms to achieve the standard form Ax + By = C.
By being mindful of these common mistakes, you can ensure accurate conversions and improve your understanding of linear equations.
Conclusion and Next Steps

Converting linear equations from slope-intercept form to standard form is a crucial skill in mathematics. By following the steps outlined in this article, you can easily convert any linear equation to standard form. Remember to practice regularly and apply your knowledge to real-world problems.
Now that you've learned how to convert Y = 3x + 4 to standard form, try practicing with other linear equations. You can also explore more advanced topics, such as solving systems of equations and graphing linear equations.
What is the standard form of a linear equation?
+The standard form of a linear equation is Ax + By = C, where A, B, and C are constants.
Why is it important to convert linear equations to standard form?
+Converting linear equations to standard form is essential for graphing, finding the x-intercept, and solving systems of equations.
What are some common mistakes to avoid when converting to standard form?
+Common mistakes include forgetting to isolate variables, failing to move constants, and not rearranging terms to achieve the standard form Ax + By = C.
We hope this article has helped you understand the process of converting Y = 3x + 4 to standard form. If you have any questions or comments, please feel free to share them below.