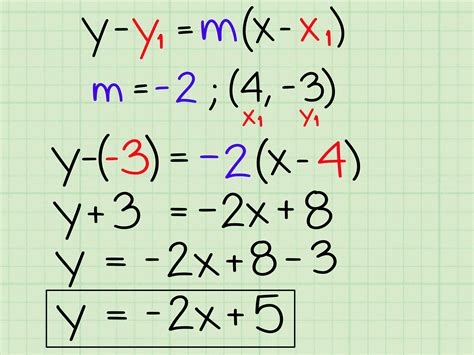3 Steps To Convert 3x 9 In Slope Intercept Form

Quick Links :

Converting linear equations to slope-intercept form is a fundamental concept in mathematics, particularly in algebra. The slope-intercept form, y = mx + b, is a widely used format for representing linear equations, where m represents the slope of the line and b is the y-intercept. In this article, we will delve into the process of converting the linear equation 3x = 9 into slope-intercept form, which involves three straightforward steps.

Step 1: Understanding the Equation

The first step is to understand the given equation, which in this case is 3x = 9. This equation represents a linear relationship between x and y. However, to convert it into slope-intercept form, we need to isolate y.

Isolating y

To isolate y, we should rearrange the equation to solve for y. However, the given equation does not explicitly have a y variable. We can imply that the equation is 3x - y = -9, assuming y is the dependent variable. By adding y to both sides of the equation, we get:

3x = y + 9

Step 2: Isolating y Further

To isolate y further, we subtract 9 from both sides of the equation:

3x - 9 = y

Now, the equation is in a format where y is isolated, but it's not yet in slope-intercept form.

Moving Towards Slope-Intercept Form

To express the equation in slope-intercept form, we need to factor out the coefficient of x, which in this case is 1/3, since we want the x term to be multiplied by a coefficient of 1. However, our equation is 3x - 9 = y, and we aim to rearrange it to match the slope-intercept form as closely as possible.

Step 3: Finalizing Slope-Intercept Form

Given that our equation is 3x - 9 = y, we can rearrange it to resemble slope-intercept form by rewriting it as:

y = 3x - 9

This equation is now in slope-intercept form, with m (slope) being 3 and b (y-intercept) being -9.

In this three-step process, we took an equation that initially did not resemble slope-intercept form and converted it to y = mx + b, where m is the slope and b is the y-intercept. Understanding the steps to convert linear equations to slope-intercept form is crucial in solving and graphing linear equations in mathematics.

Additional Insights

Converting linear equations to slope-intercept form can help in quickly identifying key features of the line, such as its slope and y-intercept. These features are essential in graphing the line and solving systems of linear equations.

Visualizing the Equation

Visualizing the equation y = 3x - 9 can be done by plotting two points on a coordinate plane and drawing a line through them. Since the slope is 3, for every one unit increase in x, y increases by three units. The y-intercept is -9, so the line crosses the y-axis at (0, -9).

Real-World Applications

Linear equations in slope-intercept form have numerous real-world applications, including physics, engineering, economics, and computer science. They are used to model real-world phenomena, make predictions, and solve problems.

Example Applications

For instance, the equation y = 3x - 9 can represent the relationship between the cost of producing x units of a product and the total cost y. In this case, the slope represents the cost per unit, and the y-intercept represents the fixed cost.

In conclusion, converting linear equations to slope-intercept form is a fundamental skill in mathematics and has numerous real-world applications. By understanding the three steps involved in converting 3x = 9 to slope-intercept form, individuals can develop a deeper appreciation for linear equations and their uses in various fields.

If you have any questions or need further clarification on converting linear equations to slope-intercept form, please share your thoughts in the comments below.