Unlocking the Secrets of Standard Form: A Comprehensive Guide

Standard form is a fundamental concept in mathematics, representing a way of expressing numbers, equations, and expressions in a compact and uniform manner. Mastering standard form is essential for simplifying complex calculations, solving problems, and communicating mathematical ideas effectively. In this article, we will delve into the world of standard form, exploring its definition, benefits, and applications, as well as providing practical tips and examples to help you become a proficient user of this powerful tool.
What is Standard Form?
Standard form, also known as scientific notation, is a way of expressing numbers in a compact form using powers of 10. It is commonly used in mathematics, physics, engineering, and other scientific fields to represent very large or very small numbers in a concise and readable format. Standard form consists of a number between 1 and 10, multiplied by a power of 10, which indicates the number's magnitude. For example, the number 456 can be written in standard form as 4.56 × 10^2.
Benefits of Standard Form
The use of standard form offers several benefits, including:
- Simplifying complex calculations: Standard form allows you to perform calculations with very large or very small numbers more easily, reducing the risk of errors and making it easier to visualize the results.
- Improving readability: Standard form makes it easier to read and compare numbers, especially when dealing with very large or very small values.
- Enhancing communication: Standard form provides a universal language for expressing numbers, making it easier to communicate mathematical ideas and results across different fields and disciplines.
5 Ways to Master Standard Form
Mastering standard form requires practice, patience, and a deep understanding of its underlying principles. Here are five ways to help you become proficient in standard form:
1. Understanding the Basics
To master standard form, you need to understand the basics of exponents and powers of 10. Start by reviewing the rules of exponents, including the product rule, quotient rule, and power rule. Practice converting numbers between standard form and ordinary notation, and learn to recognize the patterns and relationships between different powers of 10.
2. Practicing Conversions
Practice is key to mastering standard form. Start by converting simple numbers between standard form and ordinary notation, and gradually move on to more complex conversions. Use online resources, worksheets, or practice exercises to reinforce your understanding and build your skills.
3. Using Real-World Examples
Standard form is not just a theoretical concept; it has numerous practical applications in science, engineering, and everyday life. Use real-world examples to illustrate the power and versatility of standard form, such as calculating the distance to the moon, the size of atoms, or the speed of light.
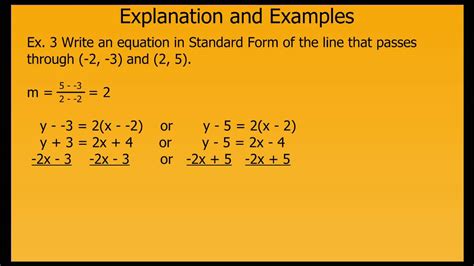
4. Applying Standard Form to Equations
Standard form is not limited to simple numbers; it can also be applied to equations and expressions. Learn to manipulate equations in standard form, using techniques such as multiplying and dividing by powers of 10, and simplifying expressions by combining like terms.
5. Solving Problems with Standard Form
The ultimate test of your mastery of standard form is applying it to solve real-world problems. Practice solving problems that involve standard form, such as calculating the area of a large rectangle, the volume of a container, or the distance between two cities.
Common Mistakes to Avoid
When working with standard form, there are several common mistakes to avoid:
- Inconsistent notation: Make sure to use consistent notation when expressing numbers in standard form, using the correct number of significant figures and powers of 10.
- Incorrect conversions: Double-check your conversions between standard form and ordinary notation, ensuring that you are using the correct powers of 10 and significant figures.
- Insufficient practice: Don't assume that you can master standard form overnight; practice regularly to build your skills and reinforce your understanding.
Conclusion: Mastering the Equation
Mastering standard form is a journey that requires patience, practice, and dedication. By understanding the basics, practicing conversions, using real-world examples, applying standard form to equations, and solving problems, you can unlock the secrets of standard form and become a proficient user of this powerful tool. Remember to avoid common mistakes, stay consistent, and practice regularly to reinforce your skills. With time and effort, you will become a master of standard form, able to tackle even the most complex calculations with ease and confidence.
What is the main benefit of using standard form?
+The main benefit of using standard form is that it simplifies complex calculations, making it easier to work with very large or very small numbers.
How do I convert a number from ordinary notation to standard form?
+To convert a number from ordinary notation to standard form, move the decimal point to the left or right until you have a number between 1 and 10, and then multiply by the corresponding power of 10.
What is the difference between standard form and scientific notation?
+Standard form and scientific notation are often used interchangeably, but technically, scientific notation refers to a specific notation system used in science and engineering, while standard form is a more general term that encompasses various notation systems.