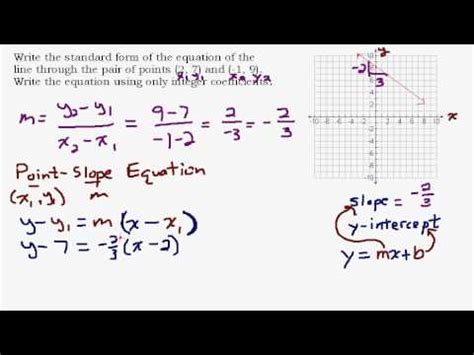Find Standard Form Equation With Two Points Calculator

Quick Links :

Finding the standard form equation of a line is a fundamental concept in mathematics, particularly in algebra and geometry. The standard form equation of a line is given by Ax + By = C, where A, B, and C are constants. If you have two points on the line, you can easily find the equation of the line using a simple formula.

In this article, we will discuss how to find the standard form equation of a line using two points calculator. We will also explain the steps involved in the process and provide examples to illustrate the concept.

Why is the Standard Form Equation Important?

The standard form equation of a line is important because it provides a unique and compact way to represent a line. It is useful in various mathematical and real-world applications, such as graphing lines, finding the slope and y-intercept of a line, and solving systems of linear equations.

Benefits of Using a Two Points Calculator

Using a two points calculator to find the standard form equation of a line has several benefits. Firstly, it saves time and effort, as you don't need to manually calculate the slope and y-intercept of the line. Secondly, it reduces the risk of errors, as the calculator provides accurate results. Finally, it helps you to visualize the line and its equation, making it easier to understand and work with.

How to Find the Standard Form Equation Using Two Points Calculator

To find the standard form equation of a line using two points calculator, follow these steps:

1. Enter the coordinates of the two points on the line. For example, let's say the two points are (2,3) and (4,5).
2. Calculate the slope of the line using the formula: m = (y2 - y1) / (x2 - x1), where (x1, y1) and (x2, y2) are the coordinates of the two points.
3. Calculate the y-intercept of the line using the formula: b = y1 - m(x1), where m is the slope and (x1, y1) is one of the points on the line.
4. Write the standard form equation of the line using the slope and y-intercept. The equation will be in the form Ax + By = C, where A and B are constants.

Example: Finding the Standard Form Equation Using Two Points Calculator

Suppose we have two points on the line: (2,3) and (4,5). To find the standard form equation of the line, we can use a two points calculator.

1. Enter the coordinates of the two points: (2,3) and (4,5).
2. Calculate the slope of the line: m = (5 - 3) / (4 - 2) = 2 / 2 = 1.
3. Calculate the y-intercept of the line: b = 3 - 1(2) = 3 - 2 = 1.
4. Write the standard form equation of the line: x + y = 5.

Advantages of Using a Two Points Calculator

Using a two points calculator to find the standard form equation of a line has several advantages. Some of the benefits include:

• Saves time and effort
• Reduces the risk of errors
• Helps to visualize the line and its equation
• Provides accurate results

Common Applications of the Standard Form Equation

The standard form equation of a line has several applications in mathematics and real-world problems. Some of the common applications include:

• Graphing lines
• Finding the slope and y-intercept of a line
• Solving systems of linear equations
• Modeling real-world problems, such as the cost of goods and the slope of a roof.

Conclusion

Finding the standard form equation of a line using two points calculator is a simple and efficient process. By following the steps outlined in this article, you can easily find the equation of a line using two points. The standard form equation of a line has several applications in mathematics and real-world problems, making it an important concept to understand.

FAQs

We hope this article has helped you understand how to find the standard form equation of a line using two points calculator. If you have any questions or need further clarification, please don't hesitate to ask.