Simplify Any Ratio: 5 Easy Steps To Fraction Form

Quick Links :

Simplifying ratios can be a daunting task, especially when dealing with complex fractions. However, with a clear understanding of the process, anyone can simplify any ratio to its simplest fraction form. In this article, we will outline 5 easy steps to simplify any ratio, making it easier to work with and understand.

Step 1: Write the Ratio as a Fraction To simplify a ratio, start by writing it as a fraction. A ratio is typically written in the form of a:b, where a and b are the two quantities being compared. To convert this to a fraction, simply place the first quantity (a) over the second quantity (b), like this: a/b.

For example, if we have the ratio 3:4, we can write it as a fraction like this: 3/4.

Understanding Equivalent Ratios

Before we dive into the simplification process, it's essential to understand the concept of equivalent ratios. Equivalent ratios are ratios that have the same value, even if they are written differently. For example, the ratios 1:2, 2:4, and 3:6 are all equivalent because they represent the same relationship between the two quantities.

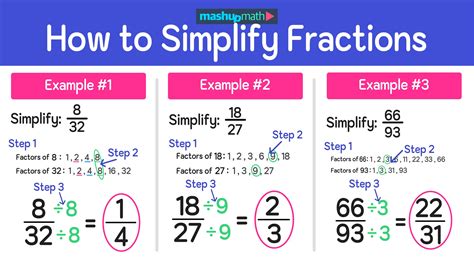
Step 2: Find the Greatest Common Divisor (GCD) The next step is to find the greatest common divisor (GCD) of the two numbers in the fraction. The GCD is the largest number that divides both numbers evenly. To find the GCD, you can use a variety of methods, such as listing the factors of each number or using a calculator.

For example, if we have the fraction 12/18, we can find the GCD by listing the factors of each number:

Factors of 12: 1, 2, 3, 4, 6, 12 Factors of 18: 1, 2, 3, 6, 9, 18

The GCD of 12 and 18 is 6.

What is the GCD Used For?

The GCD is used to simplify the fraction by dividing both numbers by the GCD. This reduces the fraction to its simplest form.

Step 3: Divide Both Numbers by the GCD Once you have found the GCD, divide both numbers in the fraction by the GCD. This will simplify the fraction.

Using the example from Step 2, we can divide both numbers by the GCD (6):

12 ÷ 6 = 2 18 ÷ 6 = 3

The simplified fraction is 2/3.

Step 4: Check for Further Simplification After simplifying the fraction, check to see if it can be further simplified. This involves finding the GCD of the new numbers and dividing both numbers by the GCD.

Using the example from Step 3, we can check if the fraction 2/3 can be further simplified:

GCD of 2 and 3: 1

Since the GCD is 1, the fraction 2/3 cannot be further simplified.

Step 5: Write the Simplified Ratio The final step is to write the simplified ratio as a fraction. This is the simplest form of the original ratio.

Using the example from Step 3, we can write the simplified ratio as a fraction:

2/3

This is the simplest form of the original ratio 12:18.

Real-World Applications of Simplifying Ratios

Simplifying ratios has many real-world applications, from cooking and construction to science and engineering. By simplifying ratios, we can make complex calculations easier to understand and work with.

For example, in cooking, simplifying ratios can help us scale recipes up or down. If a recipe calls for a ratio of 2:3 of flour to sugar, we can simplify this ratio to 1:1.5, making it easier to adjust the quantities.

In conclusion, simplifying any ratio to its simplest fraction form is a straightforward process that involves writing the ratio as a fraction, finding the GCD, dividing both numbers by the GCD, checking for further simplification, and writing the simplified ratio. By following these 5 easy steps, you can simplify any ratio and make complex calculations easier to understand and work with.