5 Ways To Write An Ellipse Equation In Standard Form

Quick Links :

Writing an ellipse equation in standard form can be a challenging task, especially for those who are new to mathematics. However, with the right approach and techniques, it can be made easier. In this article, we will explore five ways to write an ellipse equation in standard form.

What is an Ellipse Equation?

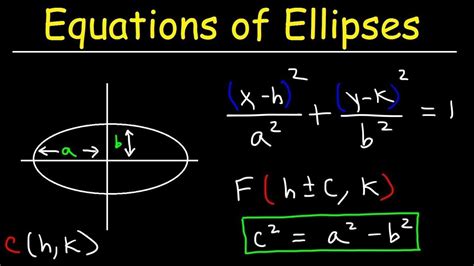
An ellipse equation is a mathematical equation that represents an ellipse, which is a closed curve on a plane surrounding two focal points such that the sum of the distances to the two focal points is constant. The standard form of an ellipse equation is (x-h)^2/a^2 + (y-k)^2/b^2 = 1, where (h,k) is the center of the ellipse, and a and b are the lengths of the semi-major and semi-minor axes, respectively.

Method 1: Using the Center and Axes Lengths

One way to write an ellipse equation in standard form is to use the center and axes lengths. If you know the center (h,k) and the lengths of the semi-major and semi-minor axes (a and b), you can plug these values into the standard form equation. For example, if the center is (2,3) and the axes lengths are 4 and 5, the equation would be (x-2)^2/4^2 + (y-3)^2/5^2 = 1.

Example:

Find the equation of an ellipse with center (1,2) and axes lengths 3 and 4.

Solution: Plug the values into the standard form equation: (x-1)^2/3^2 + (y-2)^2/4^2 = 1.

Method 2: Using the Foci and Major Axis Length

Another way to write an ellipse equation in standard form is to use the foci and major axis length. If you know the foci (F1 and F2) and the major axis length (2a), you can find the center and axes lengths. The center is the midpoint of the foci, and the axes lengths can be found using the distance formula. For example, if the foci are (-2,0) and (2,0) and the major axis length is 10, the center is (0,0) and the axes lengths are 5 and 3.

Example:

Find the equation of an ellipse with foci (1,1) and (-1,1) and major axis length 8.

Solution: Find the center: (1+(-1))/2 = 0, (1+1)/2 = 1. Find the axes lengths: distance between foci = 2, major axis length = 8, so a = 4, b = √(4^2 - 1^2) = √15.

Method 3: Using the Graph

If you have the graph of an ellipse, you can write the equation in standard form by identifying the center, foci, and axes lengths. The center is the point where the major and minor axes intersect, and the foci are the points on the major axis that are equidistant from the center. The axes lengths can be found by measuring the distances from the center to the vertices.

Example:

Find the equation of an ellipse with center (2,1), foci (1,1) and (3,1), and major axis length 6.

Solution: Find the axes lengths: distance between foci = 2, major axis length = 6, so a = 3, b = √(3^2 - 1^2) = √8.

Method 4: Using the Parametric Equations

Parametric equations are a set of equations that define a curve in terms of a parameter. Ellipse parametric equations are x = a cos(t) + h, y = b sin(t) + k, where (h,k) is the center, a and b are the axes lengths, and t is the parameter.

Example:

Find the equation of an ellipse with center (1,2), axes lengths 4 and 5, and parametric equations x = 4 cos(t) + 1, y = 5 sin(t) + 2.

Solution: Plug the parametric equations into the standard form equation: (x-1)^2/4^2 + (y-2)^2/5^2 = 1.

Method 5: Using the General Form

The general form of an ellipse equation is Ax^2 + Bxy + Cy^2 + Dx + Ey + F = 0. To write an ellipse equation in standard form, you need to complete the square and rearrange the terms.

Example:

Find the equation of an ellipse with general form 4x^2 + 9y^2 - 8x - 18y + 13 = 0.

Solution: Complete the square and rearrange the terms: (x-1)^2/4 + (y-1)^2/9 = 1.

Now that you have learned five ways to write an ellipse equation in standard form, you can practice and apply these methods to different problems. Remember to identify the center, axes lengths, and foci, and use the parametric equations or general form when necessary.