Write An Equation In Point Slope Form Easily

Quick Links :

Writing an equation in point-slope form is a fundamental concept in algebra and geometry. It's a powerful tool to find the equation of a line when you know a point on the line and the slope. In this article, we'll explore the concept of point-slope form, its significance, and provide a step-by-step guide on how to write an equation in point-slope form easily.

What is Point-Slope Form?

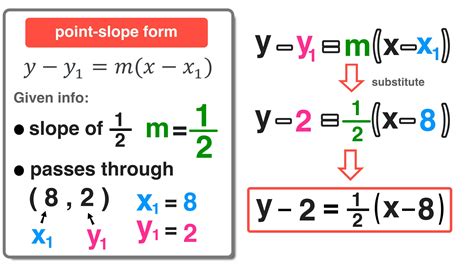
Point-slope form is a way to express the equation of a line in terms of a point on the line and the slope of the line. The general formula for point-slope form is:

y - y1 = m(x - x1)

where (x1, y1) is a point on the line, and m is the slope of the line.

Understanding the Components of Point-Slope Form

To write an equation in point-slope form, you need to understand the components involved:

• Point (x1, y1): This is a point on the line. You can choose any point on the line, but it's often easier to use the coordinates of a point that's easily accessible, such as an x-intercept or a y-intercept.
• Slope (m): The slope of the line is a measure of how steep the line is. It's calculated by finding the ratio of the vertical change (rise) to the horizontal change (run).

Steps to Write an Equation in Point-Slope Form

Here's a step-by-step guide to writing an equation in point-slope form:

1. Choose a point on the line: Select a point on the line that you're interested in. Make sure you have the coordinates of this point, which we'll call (x1, y1).
2. Find the slope of the line: Calculate the slope of the line using the formula m = (y2 - y1) / (x2 - x1), where (x2, y2) is another point on the line.
3. Plug in the values: Substitute the values of the point (x1, y1) and the slope (m) into the point-slope form formula: y - y1 = m(x - x1).
4. Simplify the equation: Simplify the equation by combining like terms and rearranging the variables.

Examples and Applications

Here are some examples to illustrate the concept of point-slope form:

• Example 1: Find the equation of the line that passes through the point (2, 3) and has a slope of 2. Using the point-slope form formula, we get: y - 3 = 2(x - 2). Simplifying the equation, we get: y = 2x - 1.
• Example 2: Find the equation of the line that passes through the points (1, 2) and (3, 4). First, we need to find the slope of the line using the slope formula: m = (4 - 2) / (3 - 1) = 1. Then, we can use the point-slope form formula with either point: y - 2 = 1(x - 1). Simplifying the equation, we get: y = x + 1.

Benefits of Point-Slope Form

Point-slope form has several benefits, including:

• Easy to use: Point-slope form is a straightforward and easy-to-use formula for finding the equation of a line.
• Flexibility: You can use point-slope form with any point on the line and the slope, making it a versatile tool.
• Powerful: Point-slope form can be used to find the equation of a line in various contexts, including algebra, geometry, and physics.

Common Mistakes to Avoid

When working with point-slope form, be sure to avoid the following common mistakes:

• Incorrectly identifying the point and slope: Double-check that you've correctly identified the point and slope of the line.
• Misusing the formula: Make sure you're using the correct formula and plugging in the correct values.
• Not simplifying the equation: Take the time to simplify the equation to ensure it's in the most straightforward form.

Conclusion and Final Thoughts

Writing an equation in point-slope form is a fundamental skill in algebra and geometry. By understanding the components involved and following the step-by-step guide, you can easily write an equation in point-slope form. Remember to avoid common mistakes and take the time to simplify the equation. With practice, you'll become proficient in using point-slope form to find the equation of a line.

What's Next?

Now that you've learned about point-slope form, try applying it to different problems and scenarios. Practice using different points and slopes to find the equation of a line. You can also explore other forms of linear equations, such as slope-intercept form and standard form.

If you have any questions or need further clarification on point-slope form, feel free to ask in the comments below. Share this article with your friends and classmates who may benefit from learning about point-slope form.