1 Simple Way To Write 25 As A Fraction

Quick Links :

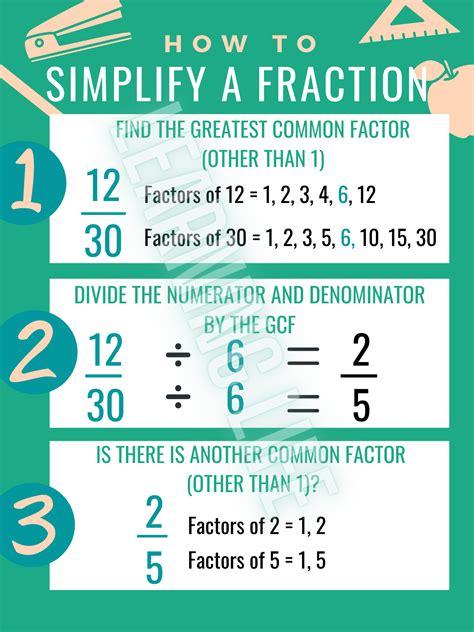
Expressing numbers as fractions can be a fascinating mathematical exercise, offering insights into the underlying structure of numbers and their relationships. The number 25, a square of 5, presents a straightforward case when considering its representation as a fraction. However, what if we aim to express 25 in a way that highlights its unique properties or simplifies its form, perhaps making it more palatable for mathematical manipulations or educational purposes?

Let's explore a simple yet insightful way to represent 25 as a fraction, delving into the mathematical reasoning and significance behind such a representation.

The Direct Approach

At its core, 25 can be expressed as a fraction in its most simplified form by considering it as twenty-five wholes divided by one whole. Mathematically, this is represented as:

25/1

This representation, although not particularly revealing, serves as a baseline for understanding 25's fractional form. It's a straightforward expression that aligns with the definition of a fraction, where the numerator (25) is the number of equal parts, and the denominator (1) is the total number of parts that make up the whole.

Exploring Alternatives

To find alternative fractional representations of 25, we need to identify other numbers that, when divided into 25, result in a whole number quotient. Essentially, we're looking for factors of 25.

The factors of 25 are 1, 5, and 25. This means that 25 can also be expressed as:

• 5/1, highlighting the relationship between 5 and 25, where 25 is five times 5.
• 25/5, showing that 25 divided by 5 equals 5, emphasizing the square relationship between 5 and 25.

However, to make the representation more intriguing, let's explore how 25 can be expressed as a fraction in a way that utilizes its square root property or offers a different perspective.

Utilizing Square Roots

Since 25 is the square of 5 (5^2 = 25), we can express 25 in terms of its square root, albeit indirectly, through fractional representation. This leads us to consider:

√25/√1

Given that √25 = 5 and √1 = 1, this representation simplifies to 5/1, which is essentially the same as our initial expression of 25/1 but now framed in terms of square roots.

A Fraction with a Common Factor

Another approach to express 25 as a fraction involves finding a common factor that can be divided into both the numerator and the denominator. A simple example is using the factor 5:

50/2

Here, both 50 and 2 are divisible by 5, but this doesn't necessarily simplify to a form that reveals anything new about 25. Instead, it's a demonstration of how fractions can be manipulated through multiplication and division by the same number.

Practical Applications

Expressing numbers like 25 as fractions can have various practical applications, especially in mathematical education. It helps in:

• Understanding Place Value: Expressing numbers in different forms can aid in understanding their place value and how numbers relate to each other.
• Simplifying Calculations: In some mathematical operations, expressing numbers as fractions can simplify calculations, especially when dealing with proportions or percentages.
• Mathematical Creativity: It encourages thinking outside the box and exploring the properties of numbers, which is crucial for problem-solving and mathematical creativity.

Engaging with the Topic

Expressing 25 as a fraction, although straightforward, can lead to deeper explorations of number properties, mathematical relationships, and educational strategies. We invite you to share your thoughts on how numbers can be creatively represented as fractions and what insights such representations can offer.

Have you encountered a unique way to express a common number as a fraction? How do you think fractional representations can enhance our understanding of mathematics?

FAQ Section