Simplifying Radicals: What Is Simplest Radical Form

Quick Links :

Simplifying radicals is a fundamental concept in mathematics, particularly in algebra and geometry. It involves expressing a radical expression in its simplest form, which is essential for solving equations, graphing functions, and performing various mathematical operations. In this article, we will delve into the world of radicals, explore the concept of simplest radical form, and provide practical examples to illustrate the simplification process.

What Are Radicals?

Radicals, also known as roots, are mathematical expressions that represent a number raised to a fractional power. The most common type of radical is the square root, denoted by the symbol √. For example, √16 represents the number that, when multiplied by itself, equals 16. Radicals can be used to simplify complex expressions, solve equations, and perform various mathematical operations.

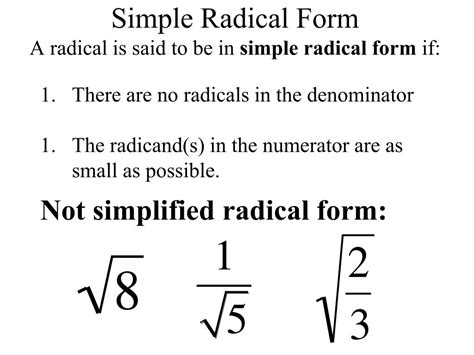
What Is Simplest Radical Form?

Simplest radical form is a mathematical expression in which the radical is simplified to its most basic form. This involves removing any perfect squares or perfect cubes from the radical, leaving only the remaining factors. For example, √16 can be simplified to 4, since 4 is the square root of 16. Similarly, √(x^2 + 4) can be simplified to x + 2, since x + 2 is the square root of x^2 + 4.

Why Is Simplest Radical Form Important?

Simplifying radicals to their simplest form is essential for several reasons:

• Easier calculations: Simplified radicals make calculations easier, as they eliminate the need to work with complex expressions.
• Improved accuracy: Simplifying radicals reduces the likelihood of errors, as it eliminates the need to perform complex calculations.
• Clearer understanding: Simplified radicals provide a clearer understanding of mathematical concepts, making it easier to visualize and analyze problems.

How to Simplify Radicals

Simplifying radicals involves a few simple steps:

1. Factor the radicand: Factor the expression inside the radical into its prime factors.
2. Identify perfect squares or perfect cubes: Identify any perfect squares or perfect cubes within the radicand.
3. Remove perfect squares or perfect cubes: Remove any perfect squares or perfect cubes from the radicand, leaving only the remaining factors.
4. Simplify the expression: Simplify the resulting expression, if possible.

Examples of Simplifying Radicals

Here are some examples of simplifying radicals:

• √16 = √(4^2) = 4
• √(x^2 + 4) = √(x + 2)^2 = x + 2
• √(2x^3) = √(2x^2) * √x = x√(2x)

Common Mistakes When Simplifying Radicals

When simplifying radicals, it's essential to avoid common mistakes, such as:

• Forgetting to factor the radicand: Failing to factor the radicand can lead to incorrect simplifications.
• Missing perfect squares or perfect cubes: Failing to identify perfect squares or perfect cubes can lead to incomplete simplifications.
• Incorrectly simplifying the expression: Failing to simplify the expression correctly can lead to incorrect results.

Real-World Applications of Simplifying Radicals

Simplifying radicals has numerous real-world applications, including:

• Physics and engineering: Simplifying radicals is essential for solving problems in physics and engineering, particularly in mechanics and electromagnetism.
• Computer science: Simplifying radicals is used in computer science, particularly in algorithms and data analysis.
• Finance: Simplifying radicals is used in finance, particularly in calculating interest rates and investment returns.

Conclusion

Simplifying radicals is a fundamental concept in mathematics that has numerous real-world applications. By understanding the concept of simplest radical form and following the steps to simplify radicals, you can improve your mathematical skills and solve complex problems with ease. Remember to avoid common mistakes and apply the concept to real-world problems.

We hope this article has provided you with a comprehensive understanding of simplifying radicals and their importance in mathematics. If you have any questions or comments, please feel free to share them below.