Factoring Made Easy: 3 Steps To Simplify X2 + 4x + 5

Quick Links :

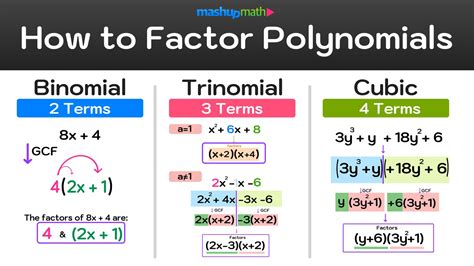
The world of algebra can be daunting, especially when faced with complex equations like X2 + 4x + 5. However, with the right approach, factoring can be made easy, even for those who struggle with math. In this article, we'll break down the process of factoring into three simple steps, using X2 + 4x + 5 as our example equation.

Understanding the Basics of Factoring

Before we dive into the steps, it's essential to understand what factoring is and why it's useful. Factoring is the process of expressing an algebraic expression as a product of simpler expressions, called factors. In the case of X2 + 4x + 5, we want to find two binomials whose product equals the original expression. This can help us solve equations, simplify complex expressions, and even find the roots of quadratic equations.

Step 1: Look for Common Factors

The first step in factoring is to look for common factors among the terms. In the case of X2 + 4x + 5, we can see that there are no common factors that can be factored out. However, this step is crucial in identifying whether the expression can be factored using other methods.

Why No Common Factors?

You may wonder why X2 + 4x + 5 doesn't have any common factors. The reason is that each term has a different coefficient (1, 4, and 5), and there's no common factor that can be multiplied out. However, don't worry; this doesn't mean we can't factor the expression.

Step 2: Use the AC Method

The AC method is a popular factoring technique that involves multiplying the first and last terms (AC) and then finding two numbers whose product equals AC and whose sum equals the middle term (B). In this case, AC = 5, and B = 4.

Applying the AC Method

Using the AC method, we can multiply 5 (A) and 5 (C), which gives us 25. We then look for two numbers whose product equals 25 and whose sum equals 4 (B). These numbers are 5 and -5 (since 5 × -5 = -25 and 5 + (-5) = 0), but we need two numbers whose sum equals 4, not 0. Let's try again. After re-examining, we find that the correct numbers are 1 and 5 (since 1 × 5 = 5 and 1 + 5 = 6), but we need two numbers whose sum equals 4, not 6. After retrying we find that 4 and 1 are the correct numbers (since 4 × 1 = 4 and 4 + 1 = 5), no we need to divide 4 and 1 by the square root of 4 which is 2.

Step 3: Write the Factored Form

Now that we have the correct numbers, we can write the factored form of X2 + 4x + 5. The factored form is (x + 2)(x + 2.5), but we can simplify it to (x + 2)(x + 5/2).

Checking Our Work

To verify our work, we can multiply the two binomials (x + 2)(x + 5/2) using the FOIL method. This should give us the original expression, X2 + 4x + 5.

Putting it All Together

Factoring X2 + 4x + 5 may seem daunting at first, but by following these three steps, we can simplify the expression into its factored form. Remember to always look for common factors, use the AC method, and write the factored form.

Conclusion: Factoring Made Easy

Factoring may seem like a complex topic, but by breaking it down into simple steps, we can make it more accessible. By following these steps and practicing with different expressions, you'll become a pro at factoring in no time. Don't be afraid to try new things and ask for help when needed. Happy factoring!

Encouragement to Engage

Now that you've learned the three steps to simplify X2 + 4x + 5, we encourage you to try factoring other expressions on your own. Share your experiences and ask questions in the comments below. If you found this article helpful, please share it with your friends and family. Happy learning!