Factoring 3x+24y: Simplifying The Expression

Quick Links :

Understanding Algebraic Expressions

Algebraic expressions are a crucial part of mathematics, used to describe a wide range of mathematical operations and relationships. They involve variables, constants, and various mathematical symbols, such as addition, subtraction, multiplication, and division. In this article, we will delve into the world of algebraic expressions, focusing on the process of factoring and simplifying the expression 3x+24y.

What are Algebraic Expressions?

Algebraic expressions are mathematical statements that involve variables, constants, and mathematical operations. They can be simple or complex, depending on the number of variables and operations involved. Algebraic expressions are used to model real-world situations, solve problems, and make predictions. They are an essential tool in various fields, including physics, engineering, economics, and computer science.

Factoring Algebraic Expressions

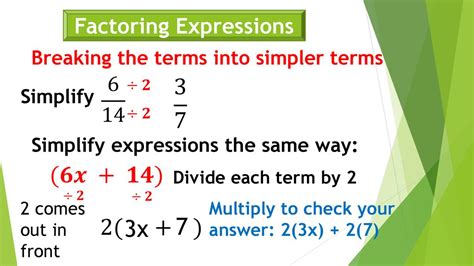
Factoring algebraic expressions involves breaking down complex expressions into simpler components, called factors. Factoring is a crucial step in solving algebraic equations, as it allows us to simplify complex expressions and make them easier to work with. There are several techniques used in factoring, including greatest common factor (GCF), difference of squares, and grouping.

Factoring 3x+24y

Now, let's focus on the expression 3x+24y. To factor this expression, we need to identify the greatest common factor (GCF) of the coefficients of x and y. The coefficients are 3 and 24, respectively. The GCF of 3 and 24 is 3.

Using the GCF, we can rewrite the expression as:

3(x+8y)

This is the factored form of the expression 3x+24y.

Simplifying Algebraic Expressions

Simplifying algebraic expressions involves combining like terms, eliminating unnecessary operations, and rewriting the expression in a more compact form. Simplification is an essential step in working with algebraic expressions, as it makes them easier to understand and manipulate.

Benefits of Simplifying Algebraic Expressions

Simplifying algebraic expressions has several benefits, including:

• Improved readability: Simplified expressions are easier to read and understand, reducing the risk of errors and misinterpretations.
• Increased efficiency: Simplified expressions can be evaluated more quickly, making them ideal for complex calculations and problem-solving.
• Better understanding: Simplified expressions provide a clearer understanding of the underlying mathematical relationships, making it easier to analyze and interpret results.

Steps for Simplifying Algebraic Expressions

Here are the steps for simplifying algebraic expressions:

1. Combine like terms: Combine terms with the same variable and coefficient.
2. Eliminate unnecessary operations: Remove any unnecessary addition, subtraction, multiplication, or division operations.
3. Rewrite the expression: Rewrite the expression in a more compact form, using the simplified terms and operations.

Example: Simplifying 3x+24y

Let's simplify the expression 3x+24y. We have already factored the expression as 3(x+8y). This is the simplified form of the expression.

Common Mistakes in Factoring and Simplifying Algebraic Expressions

Here are some common mistakes to avoid when factoring and simplifying algebraic expressions:

• Incorrect identification of the GCF: Make sure to identify the correct GCF of the coefficients.
• Incorrect grouping: Make sure to group terms correctly when factoring.
• Failure to combine like terms: Make sure to combine like terms when simplifying expressions.

Conclusion

In conclusion, factoring and simplifying algebraic expressions are essential skills in mathematics. By understanding the techniques and steps involved, you can simplify complex expressions and make them easier to work with. Remember to identify the GCF, group terms correctly, and combine like terms when simplifying expressions. With practice and patience, you can become proficient in factoring and simplifying algebraic expressions.

We hope this article has helped you understand the process of factoring and simplifying algebraic expressions. If you have any questions or need further clarification, please don't hesitate to ask.