Understanding Radical Form In Math: Simplify With 5 Steps

Quick Links :

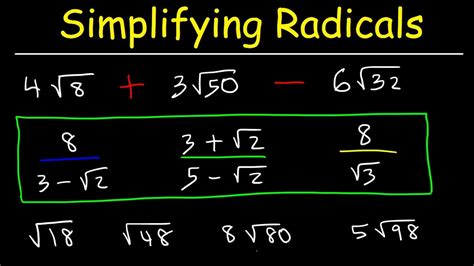
Radical forms are a fundamental part of mathematics, especially in algebra and geometry. They can seem daunting at first, but with the right approach, you can simplify even the most complex radical expressions. In this article, we'll delve into the world of radical forms, explore their importance, and provide a 5-step guide on how to simplify them.

Radical forms are used to represent the roots of numbers, and they play a crucial role in solving equations, graphing functions, and modeling real-world phenomena. However, working with radical forms can be challenging, especially when dealing with complex expressions. By understanding the principles of radical forms and learning how to simplify them, you'll become more proficient in math and improve your problem-solving skills.

What is a Radical Form in Math?

A radical form, also known as a radical expression, is a mathematical expression that contains a root, such as a square root, cube root, or nth root. The root is denoted by the symbol √ (or ∛, ∜, etc.), and it represents the operation of finding the number that, when raised to a certain power, gives the original value.

Types of Radical Forms

There are several types of radical forms, including:

• Square root (√): represents the number that, when squared, gives the original value.
• Cube root (∛): represents the number that, when cubed, gives the original value.
• Nth root (∜): represents the number that, when raised to the power of n, gives the original value.

Why Simplify Radical Forms?

Simplifying radical forms is essential in mathematics because it helps to:

• Make calculations easier and more efficient.
• Improve the accuracy of mathematical models and equations.
• Enhance problem-solving skills and critical thinking.
• Develop a deeper understanding of mathematical concepts and relationships.

5 Steps to Simplify Radical Forms

Now, let's dive into the 5-step process to simplify radical forms:

Step 1: Factor the Radical Expression

To simplify a radical form, start by factoring the expression inside the radical. This involves breaking down the expression into its prime factors.

Example: √(16x^2) = √(4 × 4 × x^2)

Step 2: Identify Perfect Squares or Perfect Cubes

Look for perfect squares or perfect cubes within the factored expression. A perfect square is a number that can be expressed as the square of an integer, such as 4 or 9. A perfect cube is a number that can be expressed as the cube of an integer, such as 8 or 27.

Example: √(4 × 4 × x^2) = √(4^2 × x^2)

Step 3: Simplify the Radical Expression

Now, simplify the radical expression by extracting the perfect squares or perfect cubes.

Example: √(4^2 × x^2) = 4x

Step 4: Check for Remaining Factors

After simplifying the radical expression, check if there are any remaining factors that can be simplified further.

Example: √(16x^2) = 4x (no remaining factors)

Step 5: Write the Simplified Radical Form

Finally, write the simplified radical form by combining the results from the previous steps.

Example: √(16x^2) = 4x

Common Mistakes to Avoid

When simplifying radical forms, avoid the following common mistakes:

• Not factoring the expression inside the radical correctly.
• Missing perfect squares or perfect cubes within the expression.
• Not checking for remaining factors after simplifying the radical expression.
• Not writing the simplified radical form correctly.

Conclusion: Mastering Radical Forms

Simplifying radical forms is an essential skill in mathematics that can help you become more proficient in algebra, geometry, and other mathematical disciplines. By following the 5-step process outlined in this article, you'll be able to simplify even the most complex radical expressions with ease. Remember to avoid common mistakes, practice regularly, and develop a deep understanding of mathematical concepts to become a master of radical forms.

We hope you found this article informative and helpful. Share your thoughts and questions in the comments below, and don't forget to share this article with your friends and colleagues who may benefit from it.