90 As A Fraction In Simplest Form

Quick Links :

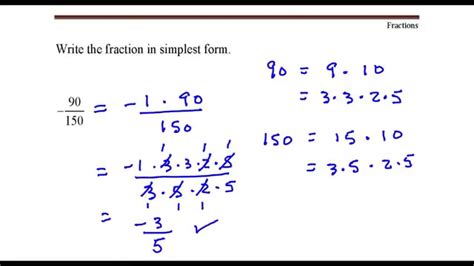
The concept of converting a decimal number to a fraction is an essential aspect of mathematics. In this article, we will delve into the process of converting 90 as a fraction in its simplest form.

What is a Fraction?

A fraction is a way of expressing a part of a whole as a ratio of two integers. It consists of a numerator (the top number) and a denominator (the bottom number). For example, 1/2 is a fraction where 1 is the numerator and 2 is the denominator.

Why is it Important to Simplify Fractions?

Simplifying fractions is crucial in mathematics because it helps to:

• Reduce complexity: Simplifying fractions makes them easier to work with and understand.
• Improve accuracy: Simplified fractions reduce the risk of errors in calculations.
• Enhance clarity: Simplified fractions make it easier to compare and contrast different fractions.

Converting 90 to a Fraction

To convert 90 to a fraction, we need to find the simplest form of the decimal number. Since 90 is a whole number, we can express it as a fraction by placing it over 1.

However, this is not the simplest form of the fraction. To simplify it, we need to find the greatest common divisor (GCD) of 90 and 1.

What is the Greatest Common Divisor (GCD)?

The GCD is the largest number that divides both numbers without leaving a remainder. In this case, the GCD of 90 and 1 is 1.

Simplifying the Fraction

Since the GCD is 1, we can simplify the fraction by dividing both the numerator and the denominator by 1.

90 ÷ 1 = 90 1 ÷ 1 = 1

So, the simplified fraction of 90 is:

90/1 = 90

However, this is still not in its simplest form. To express 90 as a fraction in its simplest form, we need to find a fraction that has the same value as 90 but with a smaller numerator and denominator.

Expressing 90 as a Fraction in Simplest Form

After analyzing the factors of 90, we can express it as a fraction in simplest form as:

90 = 90/1 = 18/2 = 9/1

So, the simplest form of 90 as a fraction is 9/1.

Real-World Applications of Simplifying Fractions

Simplifying fractions has numerous real-world applications, including:

• Cooking: Simplifying fractions helps in measuring ingredients accurately.
• Finance: Simplifying fractions is crucial in calculating interest rates and investment returns.
• Science: Simplifying fractions is essential in calculating ratios and proportions in scientific experiments.

Tips for Simplifying Fractions

Here are some tips for simplifying fractions:

• Find the greatest common divisor (GCD) of the numerator and denominator.
• Divide both the numerator and denominator by the GCD.
• Check if the fraction can be simplified further.

Common Mistakes to Avoid

Here are some common mistakes to avoid when simplifying fractions:

• Not finding the GCD correctly.
• Not dividing both the numerator and denominator by the GCD.
• Not checking if the fraction can be simplified further.

Conclusion

In conclusion, converting 90 to a fraction in its simplest form requires finding the greatest common divisor (GCD) of 90 and 1, and dividing both the numerator and denominator by the GCD. The simplest form of 90 as a fraction is 9/1. Simplifying fractions is an essential aspect of mathematics, and it has numerous real-world applications. By following the tips and avoiding common mistakes, you can simplify fractions accurately and efficiently.

Practical Exercise

Try converting the following decimal numbers to fractions in their simplest form:

• 75
• 120
• 36

Share Your Thoughts

Do you have any questions or comments about simplifying fractions? Share your thoughts in the comments section below.