Converting decimals to fractions is a fundamental concept in mathematics, and understanding how to simplify these fractions is crucial for various mathematical operations. In this article, we will delve into the process of converting the decimal 0.72 to a fraction in its simplest form.
Understanding Decimals and Fractions

Before we dive into the conversion process, it's essential to understand the basics of decimals and fractions. Decimals are a way of expressing numbers using a point to separate the whole part from the fractional part. Fractions, on the other hand, represent a part of a whole as a ratio of two numbers. The numerator represents the number of equal parts, and the denominator represents the total number of parts.
Converting Decimals to Fractions
To convert a decimal to a fraction, we need to identify the place value of the last digit. In the case of 0.72, the last digit is 2, which is in the hundredths place. This means that 0.72 can be written as 72/100.
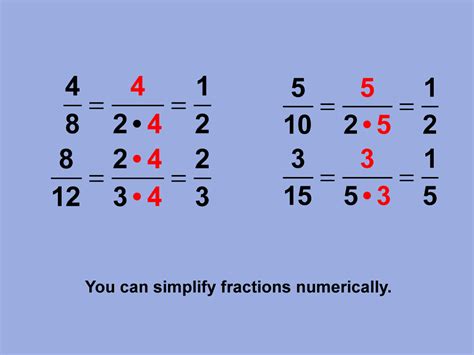
Simplifying Fractions

Simplifying fractions involves finding the greatest common divisor (GCD) of the numerator and denominator and dividing both numbers by the GCD. In the case of 72/100, the GCD is 4. Dividing both numbers by 4 gives us 18/25.
Why Simplifying Fractions is Important
Simplifying fractions is crucial in mathematics because it helps to:
- Reduce errors in calculations
- Make fractions easier to compare and order
- Simplify mathematical operations such as addition, subtraction, multiplication, and division
- Improve understanding of mathematical concepts
Real-World Applications of Simplifying Fractions

Simplifying fractions has numerous real-world applications, including:
- Cooking and recipes: Simplifying fractions helps to scale recipes up or down and ensures that ingredients are measured accurately.
- Finance: Simplifying fractions is essential in finance, where fractions are used to calculate interest rates, investment returns, and currency exchange rates.
- Science: Simplifying fractions is crucial in scientific calculations, such as measuring quantities, calculating ratios, and determining proportions.
Practical Examples of Simplifying Fractions
Here are some practical examples of simplifying fractions:
- 1/2 = 2/4 = 3/6 (all these fractions are equivalent, but 1/2 is the simplest form)
- 3/4 = 6/8 = 9/12 (all these fractions are equivalent, but 3/4 is the simplest form)
- 2/3 = 4/6 = 6/9 (all these fractions are equivalent, but 2/3 is the simplest form)
Conclusion and Final Thoughts

In conclusion, converting decimals to fractions and simplifying them is an essential mathematical concept. By understanding how to simplify fractions, we can improve our mathematical accuracy, simplify calculations, and develop a deeper understanding of mathematical concepts. We hope this article has provided you with a comprehensive understanding of how to convert 0.72 to a fraction in its simplest form.
Take Action and Engage
We encourage you to practice converting decimals to fractions and simplifying them. Try converting different decimals to fractions and simplifying them to their simplest form. Share your experiences and examples in the comments below. If you have any questions or need further clarification, please don't hesitate to ask.
What is the simplest form of the fraction 0.72?
+The simplest form of the fraction 0.72 is 18/25.
Why is simplifying fractions important?
+Simplifying fractions is important because it helps to reduce errors in calculations, makes fractions easier to compare and order, simplifies mathematical operations, and improves understanding of mathematical concepts.
What are some real-world applications of simplifying fractions?
+Simplifying fractions has numerous real-world applications, including cooking and recipes, finance, and science.