5 Easy Steps To Convert Vertex To Standard Form

Quick Links :

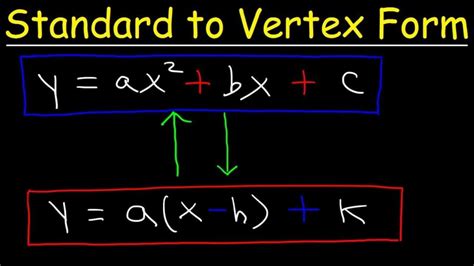
Converting a quadratic function from vertex form to standard form is a crucial skill in algebra and mathematics. The vertex form of a quadratic function is given by f(x) = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. However, there are situations where you need to express the quadratic function in the standard form, which is f(x) = ax^2 + bx + c. In this article, we will explore the steps to convert vertex form to standard form.

Why is Converting Vertex Form to Standard Form Important?

Converting vertex form to standard form is essential in various mathematical contexts. For instance, when solving quadratic equations or inequalities, it is often necessary to express the quadratic function in standard form. Moreover, many algebraic manipulations and techniques, such as factoring, completing the square, or using the quadratic formula, require the quadratic function to be in standard form.

Step 1: Understand the Vertex Form

The first step is to understand the vertex form of the quadratic function. The vertex form is given by f(x) = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. The value of 'a' represents the direction and width of the parabola, while 'h' and 'k' represent the coordinates of the vertex.

Breaking Down the Vertex Form

• The value of 'a' can be positive or negative, representing the direction of the parabola. If 'a' is positive, the parabola opens upwards, and if 'a' is negative, the parabola opens downwards.
• The value of 'h' represents the x-coordinate of the vertex, which is the axis of symmetry of the parabola.
• The value of 'k' represents the y-coordinate of the vertex, which is the minimum or maximum point of the parabola.

Step 2: Expand the Vertex Form

The next step is to expand the vertex form using the algebraic identity (x - h)^2 = x^2 - 2hx + h^2. By expanding the squared term, we get f(x) = a(x^2 - 2hx + h^2) + k.

Expanding the Vertex Form

• Multiply the value of 'a' with the squared term (x - h)^2 to get ax^2 - 2ahx + ah^2.
• Add the value of 'k' to the result to get ax^2 - 2ahx + ah^2 + k.

Step 3: Simplify the Expression

Now, simplify the expression by combining like terms. The resulting expression should be in the form ax^2 + bx + c.

Simplifying the Expression

• Combine the x^2 terms to get ax^2.
• Combine the x terms to get -2ahx.
• Combine the constant terms to get ah^2 + k.

Step 4: Rearrange the Terms

Rearrange the terms to get the standard form of the quadratic function. The standard form is given by f(x) = ax^2 + bx + c.

Rearranging the Terms

• Move the x^2 term to the leftmost position.
• Move the x term to the middle position.
• Move the constant term to the rightmost position.

Step 5: Verify the Result

The final step is to verify the result by checking if the quadratic function is in standard form. The standard form should be in the form f(x) = ax^2 + bx + c.

Verifying the Result

• Check if the x^2 term is in the leftmost position.
• Check if the x term is in the middle position.
• Check if the constant term is in the rightmost position.

By following these 5 easy steps, you can convert a quadratic function from vertex form to standard form. Remember to understand the vertex form, expand the squared term, simplify the expression, rearrange the terms, and verify the result.