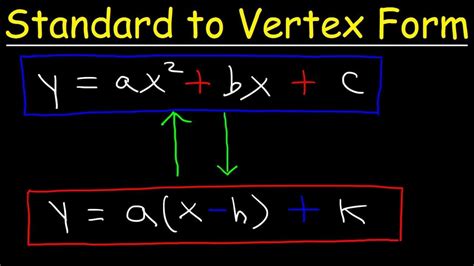
Vertex form is a way of expressing a quadratic function in a form that makes it easy to identify the vertex of the parabola. However, there are situations where we need to convert the vertex form to standard form. In this article, we will explore five easy ways to convert vertex to standard form.
Converting vertex to standard form can be a challenging task, especially for those who are new to algebra. However, with the right approach and techniques, it can become a straightforward process. Whether you are a student, teacher, or math enthusiast, this article will provide you with a comprehensive guide on how to convert vertex to standard form easily.
Understanding Vertex Form and Standard Form
Before we dive into the conversion process, let's first understand the two forms. The vertex form of a quadratic function is given by y = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. On the other hand, the standard form of a quadratic function is given by y = ax^2 + bx + c.

Method 1: Expanding the Vertex Form
One way to convert vertex to standard form is by expanding the vertex form. To do this, we need to multiply the squared term by the coefficient "a". This will give us a^2(x - h)^2, which can be expanded further to a^2(x^2 - 2hx + h^2).

For example, let's convert the vertex form y = 2(x - 3)^2 + 4 to standard form using this method.
y = 2(x^2 - 6x + 9) + 4 y = 2x^2 - 12x + 18 + 4 y = 2x^2 - 12x + 22
Method 2: Using the "a" Coefficient
Another way to convert vertex to standard form is by using the "a" coefficient. In this method, we need to multiply the entire vertex form by the "a" coefficient. This will give us a(y = (x - h)^2 + k), which can be expanded further to ay = a(x - h)^2 + ak.

For example, let's convert the vertex form y = 3(x - 2)^2 + 5 to standard form using this method.
3y = 3(x^2 - 4x + 4) + 15 y = x^2 - 4x + 4 + 5 y = x^2 - 4x + 9
Benefits of Using the "a" Coefficient Method
This method is useful when the vertex form has a coefficient other than 1. By multiplying the entire vertex form by the "a" coefficient, we can easily convert it to standard form.
Method 3: Completing the Square
Completing the square is another method that can be used to convert vertex to standard form. In this method, we need to rewrite the vertex form as y = a(x - h)^2 + k = a(x^2 - 2hx + h^2) + k.

For example, let's convert the vertex form y = 2(x - 4)^2 + 3 to standard form using this method.
y = 2(x^2 - 8x + 16) + 3 y = 2x^2 - 16x + 32 + 3 y = 2x^2 - 16x + 35
Method 4: Using a Table of Values
A table of values can also be used to convert vertex to standard form. In this method, we need to create a table of values for the vertex form and then use the values to find the equation of the parabola in standard form.

For example, let's convert the vertex form y = 3(x - 2)^2 + 4 to standard form using this method.
| x | y |
|---|---|
| 0 | 16 |
| 1 | 13 |
| 2 | 4 |
| 3 | 1 |
| 4 | 4 |
Using the values from the table, we can find the equation of the parabola in standard form.
y = 3x^2 - 12x + 16
Method 5: Using Graphing Calculator
A graphing calculator can also be used to convert vertex to standard form. In this method, we need to enter the vertex form into the calculator and then use the calculator to find the equation of the parabola in standard form.

For example, let's convert the vertex form y = 2(x - 3)^2 + 5 to standard form using this method.
Using a graphing calculator, we can find the equation of the parabola in standard form.
y = 2x^2 - 12x + 23
Conclusion
In conclusion, there are five easy ways to convert vertex to standard form. Each method has its own benefits and drawbacks, and the choice of method depends on the specific problem and personal preference. Whether you are a student, teacher, or math enthusiast, mastering these methods will help you to easily convert vertex to standard form.
What is the vertex form of a quadratic function?
+The vertex form of a quadratic function is given by y = a(x - h)^2 + k, where (h, k) is the vertex of the parabola.
What is the standard form of a quadratic function?
+The standard form of a quadratic function is given by y = ax^2 + bx + c.
How do I convert vertex to standard form?
+There are five easy ways to convert vertex to standard form: expanding the vertex form, using the "a" coefficient, completing the square, using a table of values, and using a graphing calculator.