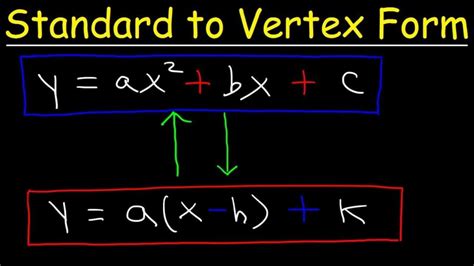
Converting a quadratic equation from vertex form to standard form is a crucial skill in algebra, and it's essential to understand the steps involved. In this article, we'll break down the process into five manageable steps, providing you with a clear understanding of how to convert vertex form to standard form.
Understanding Vertex Form and Standard Form

Before diving into the conversion process, let's briefly review the definitions of vertex form and standard form.
Vertex form is a quadratic equation in the form y = a(x - h)^2 + k, where (h, k) represents the vertex of the parabola. Standard form, on the other hand, is a quadratic equation in the form ax^2 + bx + c = 0.
Step 1: Identify the Vertex and the Value of 'a'

To begin the conversion process, identify the vertex (h, k) and the value of 'a' in the vertex form equation. The vertex will be represented as (h, k), and the value of 'a' will be the coefficient of the squared term.
Example:
Given the vertex form equation y = 2(x - 3)^2 + 4, identify the vertex and the value of 'a'.- Vertex: (3, 4)
- Value of 'a': 2
Step 2: Expand the Squared Term

Next, expand the squared term (x - h)^2 using the formula (x - h)^2 = x^2 - 2hx + h^2.
Example:
Expand the squared term (x - 3)^2:(x - 3)^2 = x^2 - 2(3)x + 3^2 = x^2 - 6x + 9
Step 3: Rewrite the Vertex Form Equation

Now, rewrite the vertex form equation using the expanded squared term and the value of 'a'.
Example:
Rewrite the vertex form equation y = 2(x - 3)^2 + 4:y = 2(x^2 - 6x + 9) + 4 = 2x^2 - 12x + 18 + 4 = 2x^2 - 12x + 22
Step 4: Rearrange the Equation to Standard Form

Rearrange the equation to standard form by moving all terms to one side of the equation.
Example:
Rearrange the equation 2x^2 - 12x + 22 to standard form:2x^2 - 12x + 22 = 0
Step 5: Simplify the Equation (Optional)

If necessary, simplify the equation by combining like terms or factoring.
Example:
No simplification needed for the equation 2x^2 - 12x + 22 = 0.By following these five steps, you can convert a quadratic equation from vertex form to standard form.
We hope this article has provided you with a clear understanding of the conversion process. If you have any questions or need further clarification, please don't hesitate to ask.
Don't forget to share this article with your friends and classmates who may benefit from it. If you have any feedback or suggestions, please leave a comment below.
What is the difference between vertex form and standard form?
+Vertex form is a quadratic equation in the form y = a(x - h)^2 + k, while standard form is a quadratic equation in the form ax^2 + bx + c = 0.
Why is it important to convert vertex form to standard form?
+Converting vertex form to standard form is important because it allows us to easily identify the vertex and other key features of the parabola, making it easier to analyze and solve problems.
Can you provide more examples of converting vertex form to standard form?
+Yes, please let us know if you'd like more examples, and we'll be happy to provide them.