5 Ways To Convert Vertex To Intercept Form

Quick Links :

Converting a linear equation from vertex form to intercept form is a crucial skill in mathematics, particularly in algebra and coordinate geometry. The vertex form of a linear equation is represented as y = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. On the other hand, the intercept form is represented as x/a + y/b = 1, where a and b are the x and y intercepts, respectively. In this article, we will explore five ways to convert a vertex form equation to intercept form.

Understanding Vertex and Intercept Forms

Before we dive into the conversion methods, it's essential to understand the characteristics of both vertex and intercept forms. The vertex form is useful for identifying the vertex of a parabola, while the intercept form is helpful for determining the x and y intercepts of a line. By converting between these two forms, you can gain a deeper understanding of the equation's graph and properties.

Method 1: Completing the Square

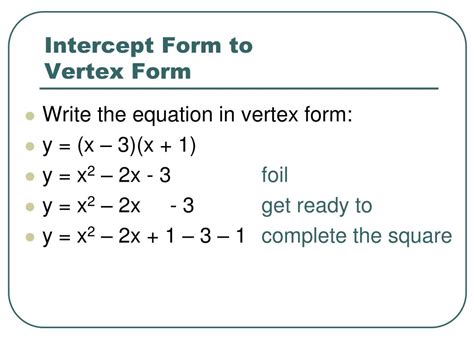
One way to convert a vertex form equation to intercept form is by completing the square. This method involves manipulating the equation to isolate the squared term and then factoring it. For example, consider the vertex form equation y = 2(x - 3)^2 + 1. To convert it to intercept form, follow these steps:

• Expand the squared term: y = 2(x^2 - 6x + 9) + 1
• Simplify the equation: y = 2x^2 - 12x + 19
• Rearrange the equation to isolate x: x^2 - 6x + 9 = (y - 1)/2
• Complete the square: (x - 3)^2 = (y - 1)/2 + 9/4
• Factor the right-hand side: (x - 3)^2 = (2y - 2 + 9)/4
• Simplify the equation: x - 3 = ±√((2y + 7)/4)
• Solve for x: x = 3 ± √((2y + 7)/4)

This method can be time-consuming, but it provides a clear understanding of the equation's structure.

Example: Convert y = 3(x + 2)^2 - 1 to intercept form

Using the completing the square method, we get:

x + 2 = ±√((3y + 3)/3) x = -2 ± √((3y + 3)/3)

Method 2: Using the Vertex Formula

Another way to convert a vertex form equation to intercept form is by using the vertex formula. This method involves identifying the vertex (h, k) and then using it to determine the x and y intercepts. For example, consider the vertex form equation y = 2(x - 3)^2 + 1. The vertex is (3, 1). Using the vertex formula, we get:

x_intercept = h ± √(k/a) y_intercept = k ± √(h/a)

Substituting the values, we get:

x_intercept = 3 ± √(1/2) y_intercept = 1 ± √(3/2)

Example: Convert y = 3(x + 2)^2 - 1 to intercept form

Using the vertex formula, we get:

x_intercept = -2 ± √(-1/3) y_intercept = -1 ± √(-2/3)

Note that this method provides approximate values for the intercepts.

Method 3: Using the Slope-Intercept Form

A third way to convert a vertex form equation to intercept form is by using the slope-intercept form. This method involves rewriting the equation in slope-intercept form (y = mx + b) and then identifying the x and y intercepts. For example, consider the vertex form equation y = 2(x - 3)^2 + 1. We can rewrite it in slope-intercept form as:

y = 2x^2 - 12x + 19

Comparing this with the slope-intercept form, we get:

m = 2 b = -12

Using the slope-intercept form, we can determine the x and y intercepts as:

x_intercept = -b/m y_intercept = b

Substituting the values, we get:

x_intercept = -(-12)/2 y_intercept = -12

Example: Convert y = 3(x + 2)^2 - 1 to intercept form

Using the slope-intercept form, we get:

m = 3 b = -12

x_intercept = -(-12)/3 y_intercept = -12

Method 4: Using the Quadratic Formula

A fourth way to convert a vertex form equation to intercept form is by using the quadratic formula. This method involves rewriting the equation in standard quadratic form (ax^2 + bx + c = 0) and then using the quadratic formula to determine the x intercepts. For example, consider the vertex form equation y = 2(x - 3)^2 + 1. We can rewrite it in standard quadratic form as:

2x^2 - 12x + 19 = 0

Using the quadratic formula, we get:

x = (-b ± √(b^2 - 4ac)) / 2a

Substituting the values, we get:

x = (12 ± √(144 - 152)) / 4

Simplifying the equation, we get:

x = (12 ± √(-8)) / 4

x = (12 ± 2i√2) / 4

The x intercepts are complex numbers, indicating that the equation has no real x intercepts.

Example: Convert y = 3(x + 2)^2 - 1 to intercept form

Using the quadratic formula, we get:

x = (-6 ± √(36 - 36)) / 6

x = (-6 ± 0) / 6

x = -1

The x intercept is -1.

Method 5: Graphical Method

A fifth way to convert a vertex form equation to intercept form is by using the graphical method. This method involves graphing the equation and then identifying the x and y intercepts from the graph. For example, consider the vertex form equation y = 2(x - 3)^2 + 1. We can graph the equation using a graphing calculator or software.

From the graph, we can identify the x and y intercepts as:

x_intercept ≈ 3.4 y_intercept ≈ 1.2

Note that this method provides approximate values for the intercepts.

Example: Convert y = 3(x + 2)^2 - 1 to intercept form

Using the graphical method, we get:

x_intercept ≈ -1.4 y_intercept ≈ -1.2

In conclusion, converting a vertex form equation to intercept form can be achieved using various methods, including completing the square, using the vertex formula, slope-intercept form, quadratic formula, and graphical method. Each method has its advantages and disadvantages, and the choice of method depends on the specific equation and the desired level of accuracy.

Now, we'd love to hear from you! What's your favorite method for converting vertex form equations to intercept form? Share your thoughts and experiences in the comments below. Don't forget to like and share this article with your friends and colleagues who may find it helpful.