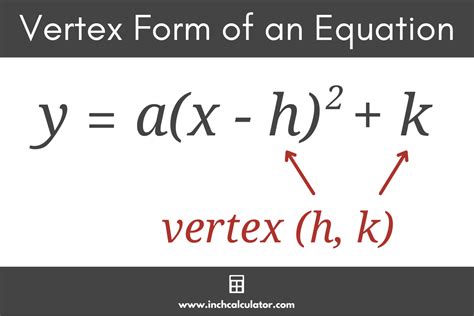
Vertex Form Calculator With Steps: Easy Quadratic Equation Solver

Quick Links :

Vertex form calculators are powerful tools that can help solve quadratic equations with ease. A quadratic equation is a polynomial equation of degree two, which means the highest power of the variable is two. These equations can be written in the general form of ax^2 + bx + c = 0, where a, b, and c are constants. One of the most useful forms of a quadratic equation is the vertex form, which is written as y = a(x - h)^2 + k.

What is the Vertex Form of a Quadratic Equation?

The vertex form of a quadratic equation provides valuable information about the parabola it represents. The vertex of a parabola is the lowest or highest point on the curve, depending on the direction it opens. In the vertex form, (h, k) represents the coordinates of the vertex. The value of 'a' determines the direction and width of the parabola. If 'a' is positive, the parabola opens upwards; if 'a' is negative, it opens downwards. The greater the absolute value of 'a', the narrower the parabola.

Benefits of Using Vertex Form Calculators

Vertex form calculators can simplify the process of solving quadratic equations by providing the vertex coordinates and the equation of the parabola in vertex form. This can be particularly useful for graphing quadratic functions and identifying key features such as the vertex, axis of symmetry, and x-intercepts.

How to Use a Vertex Form Calculator

Using a vertex form calculator is straightforward. Typically, you will need to enter the coefficients of the quadratic equation in the standard form (ax^2 + bx + c = 0). The calculator will then provide the vertex coordinates (h, k) and the equation in vertex form.

Steps to Follow

Here are the general steps to follow when using a vertex form calculator:

• Enter the coefficients a, b, and c of the quadratic equation.
• The calculator will display the vertex coordinates (h, k).
• The calculator will also display the equation in vertex form.
• Use the vertex coordinates and the equation to analyze the parabola and identify key features.

Example of Using a Vertex Form Calculator

Consider the quadratic equation x^2 + 5x + 6 = 0. To find the vertex form using a calculator, you would enter the coefficients a = 1, b = 5, and c = 6. The calculator would then display the vertex coordinates (h, k) and the equation in vertex form.

Interpreting the Results

Once you have obtained the vertex form of the quadratic equation, you can use this information to analyze the parabola. The vertex coordinates (h, k) provide the location of the vertex, while the equation in vertex form can be used to identify key features such as the axis of symmetry and x-intercepts.

Advantages of Vertex Form Calculators

Vertex form calculators offer several advantages over traditional methods of solving quadratic equations. These advantages include:

• Simplified calculations: Vertex form calculators can perform complex calculations quickly and accurately, reducing the risk of errors.
• Easy identification of key features: The vertex form provides valuable information about the parabola, making it easier to identify key features such as the vertex, axis of symmetry, and x-intercepts.
• Graphing: The vertex form is ideal for graphing quadratic functions, as it provides the vertex coordinates and the equation of the parabola.

Common Applications of Vertex Form Calculators

Vertex form calculators have a wide range of applications in mathematics, physics, engineering, and other fields. Some common applications include:

• Graphing quadratic functions
• Identifying key features of parabolas
• Solving quadratic equations
• Analyzing quadratic functions in physics and engineering

Conclusion

Vertex form calculators are powerful tools that can simplify the process of solving quadratic equations and analyzing parabolas. By providing the vertex coordinates and the equation in vertex form, these calculators can help identify key features and graph quadratic functions with ease. Whether you are a student, teacher, or professional, a vertex form calculator is an essential tool to have in your mathematical toolkit.