5 Ways To Master Vertex Form Of A Quadratic Equation

Quick Links :

Mastering the vertex form of a quadratic equation is an essential skill for any math student or enthusiast. The vertex form, also known as the "a-h-k" form, is a powerful tool for analyzing and graphing quadratic functions. In this article, we will explore five ways to master the vertex form of a quadratic equation, including understanding the basics, converting standard form to vertex form, finding the vertex, using the vertex to graph, and applying real-world examples.

Understanding the Basics of Vertex Form

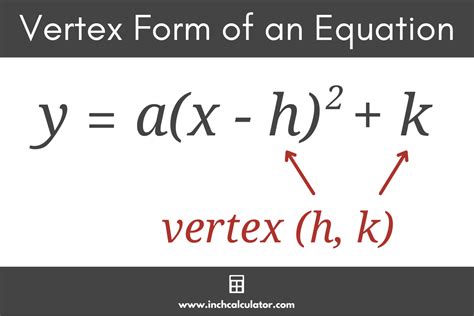
The vertex form of a quadratic equation is written as:

y = a(x - h)^2 + k

Where:

• (h, k) is the vertex of the parabola
• a is the coefficient that determines the direction and width of the parabola

To master the vertex form, it's essential to understand the role of each variable. The vertex (h, k) represents the turning point of the parabola, while the coefficient "a" determines the direction and width of the parabola.

Why is Vertex Form Important?

The vertex form is a powerful tool for analyzing and graphing quadratic functions. By identifying the vertex, you can:

• Determine the maximum or minimum value of the function
• Identify the axis of symmetry
• Graph the function with ease

Converting Standard Form to Vertex Form

To convert a quadratic equation from standard form (y = ax^2 + bx + c) to vertex form, follow these steps:

1. Complete the square: Start by dividing the coefficient of x^2 (a) and moving the constant term (c) to the right side.
2. Identify the vertex: The x-coordinate of the vertex (h) is the value that completes the square.
3. Write the vertex form: Use the values of a, h, and k to write the vertex form of the equation.

Example:

Standard form: y = x^2 + 4x + 4

Vertex form: y = (x + 2)^2

Note: To convert from standard form to vertex form, you can use the formula:

h = -b / 2a k = c - (b^2 / 4a)

Finding the Vertex

Finding the vertex is a crucial step in mastering the vertex form. To find the vertex, you can:

1. Complete the square: Use the method described above to complete the square.
2. Use the formula: Use the formula h = -b / 2a to find the x-coordinate of the vertex.
3. Identify the y-coordinate: Plug the x-coordinate of the vertex into the equation to find the y-coordinate.

Example:

y = x^2 + 4x + 4

h = -4 / 2(1) = -2 k = 4 - (4^2 / 4(1)) = 0

Vertex: (-2, 0)

Using the Vertex to Graph

Once you've found the vertex, you can use it to graph the function. Here are the steps:

1. Plot the vertex: Plot the vertex on the coordinate plane.
2. Determine the direction: Use the coefficient "a" to determine the direction of the parabola.
3. Plot the axis of symmetry: Use the x-coordinate of the vertex to plot the axis of symmetry.
4. Plot additional points: Plot additional points on either side of the axis of symmetry to complete the graph.

Example:

y = (x + 2)^2

Vertex: (-2, 0)

Direction: Upward (since a = 1)

Axis of symmetry: x = -2

Additional points: (-3, 1), (-1, 1), (0, 4)

Applying Real-World Examples

Mastering the vertex form has numerous real-world applications. Here are a few examples:

• Projectile motion: The vertex form can be used to model the trajectory of projectiles, such as a thrown ball or a launched rocket.
• Optics: The vertex form can be used to model the behavior of light as it passes through a lens or a mirror.
• Economics: The vertex form can be used to model the behavior of supply and demand curves.

By mastering the vertex form, you can develop a deeper understanding of these real-world applications and improve your problem-solving skills.

Encourage Engagement:

We hope this article has helped you master the vertex form of a quadratic equation. Whether you're a math student or enthusiast, we encourage you to practice and apply the concepts learned in this article. Share your thoughts and questions in the comments below, and don't forget to like and share this article with your friends and colleagues!

FAQ Section: