Convert Standard Form To Vertex Form Easily

Quick Links :

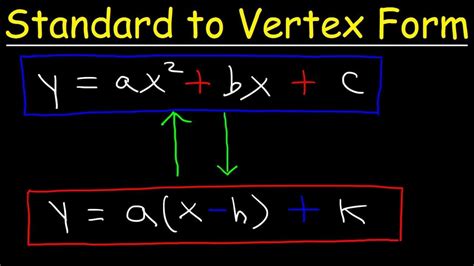
Converting a quadratic function from standard form to vertex form is a crucial skill in algebra and mathematics. The standard form of a quadratic function is f(x) = ax^2 + bx + c, where a, b, and c are constants. On the other hand, the vertex form of a quadratic function is f(x) = a(x-h)^2 + k, where (h, k) is the vertex of the parabola. In this article, we will explore the steps and methods to convert a quadratic function from standard form to vertex form easily.

Understanding the Importance of Vertex Form

Before diving into the conversion process, it's essential to understand the significance of vertex form. The vertex form of a quadratic function provides valuable information about the parabola, such as the vertex, axis of symmetry, and the direction of the parabola. The vertex form also makes it easier to graph the parabola and analyze its behavior.

Step-by-Step Conversion Process

Converting a quadratic function from standard form to vertex form involves a simple process. Here's a step-by-step guide to help you convert standard form to vertex form easily:

Step 1: Identify the Values of a, b, and c

Start by identifying the values of a, b, and c in the standard form of the quadratic function. These values are essential in determining the vertex of the parabola.

Step 2: Calculate the x-Coordinate of the Vertex

The x-coordinate of the vertex (h) can be calculated using the formula h = -b/2a. This formula is derived from the axis of symmetry of the parabola.

Step 3: Calculate the y-Coordinate of the Vertex

Once you have the x-coordinate of the vertex (h), substitute this value into the standard form of the quadratic function to find the y-coordinate (k). The y-coordinate of the vertex can be calculated using the formula k = f(h) = a(h)^2 + b(h) + c.

Step 4: Write the Quadratic Function in Vertex Form

Now that you have the values of a, h, and k, you can write the quadratic function in vertex form. The vertex form of a quadratic function is f(x) = a(x-h)^2 + k.

Example Problem

Suppose we have a quadratic function in standard form: f(x) = 2x^2 + 4x + 1. Convert this function to vertex form.

Step 1: Identify the Values of a, b, and c

a = 2, b = 4, and c = 1.

Step 2: Calculate the x-Coordinate of the Vertex

h = -b/2a = -4/(2*2) = -1.

Step 3: Calculate the y-Coordinate of the Vertex

k = f(h) = f(-1) = 2(-1)^2 + 4(-1) + 1 = 2 - 4 + 1 = -1.

Step 4: Write the Quadratic Function in Vertex Form

f(x) = 2(x-(-1))^2 + (-1) = 2(x+1)^2 - 1.

Tips and Tricks

Here are some tips and tricks to help you convert standard form to vertex form easily:

• Always identify the values of a, b, and c before starting the conversion process.
• Use the formula h = -b/2a to calculate the x-coordinate of the vertex.
• Substitute the x-coordinate of the vertex into the standard form of the quadratic function to find the y-coordinate.
• Double-check your calculations to ensure accuracy.

Conclusion

Converting a quadratic function from standard form to vertex form is a straightforward process that requires attention to detail and basic algebraic skills. By following the steps outlined in this article, you can easily convert standard form to vertex form and gain a deeper understanding of the parabola's behavior. Remember to practice, practice, practice to become proficient in converting standard form to vertex form.

We hope this article has been informative and helpful in your algebraic journey. If you have any questions or need further clarification, please don't hesitate to ask.

Take Action

• Practice converting standard form to vertex form using different quadratic functions.
• Share this article with your friends and classmates who may benefit from it.
• Leave a comment below with your thoughts, questions, or suggestions.