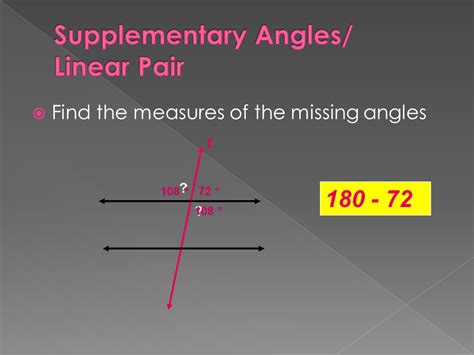
In the realm of geometry, angles play a crucial role in defining the properties of shapes and figures. Among the various types of angles, supplementary angles hold a special significance. Supplementary angles are pairs of angles that add up to 180 degrees, making them an essential concept in geometric calculations. One way to form supplementary angles is through linear pairs. In this article, we will delve into the world of linear pairs and explore five ways they form supplementary angles.
What are Linear Pairs?

A linear pair is a set of two adjacent angles that share a common vertex and lie on the same line. The key characteristic of linear pairs is that they are supplementary, meaning their measures add up to 180 degrees. Linear pairs can be formed in various ways, and understanding these formations is crucial for solving geometric problems.
1. Adjacent Angles on a Line
One of the simplest ways to form linear pairs is by creating adjacent angles on a line. When two angles share a common vertex and are adjacent to each other, they form a linear pair. For instance, consider two angles, ∠A and ∠B, that share a common vertex O and lie on the same line. Since they are adjacent and share a common vertex, ∠A and ∠B form a linear pair.
Types of Linear Pairs

Linear pairs can be classified into different types based on the orientation of the angles. The most common types of linear pairs are:
- Vertical Linear Pairs: These are linear pairs formed by two angles that are opposite each other and share a common vertex.
- Adjacent Linear Pairs: These are linear pairs formed by two angles that share a common vertex and are adjacent to each other.
- Alternate Interior Linear Pairs: These are linear pairs formed by two angles that lie on opposite sides of a transversal and share a common vertex.
2. Vertical Angles
Vertical angles are another way to form linear pairs. When two lines intersect, they form a pair of vertical angles. These angles are equal in measure and form a linear pair. For example, consider two lines that intersect at point O, forming angles ∠A and ∠B. Since ∠A and ∠B are vertical angles, they form a linear pair.
Properties of Linear Pairs

Linear pairs have several properties that make them useful in geometric calculations. Some of the key properties of linear pairs include:
- Supplementary Angles: Linear pairs are supplementary, meaning their measures add up to 180 degrees.
- Equal Measures: Vertical linear pairs have equal measures, while adjacent linear pairs have measures that add up to 180 degrees.
3. Alternate Interior Angles
Alternate interior angles are another way to form linear pairs. When a transversal intersects two parallel lines, it forms a pair of alternate interior angles. These angles are equal in measure and form a linear pair. For instance, consider a transversal that intersects two parallel lines, forming angles ∠A and ∠B. Since ∠A and ∠B are alternate interior angles, they form a linear pair.
4. Corresponding Angles
Corresponding angles are another type of linear pair. When a transversal intersects two parallel lines, it forms a pair of corresponding angles. These angles are equal in measure and form a linear pair. For example, consider a transversal that intersects two parallel lines, forming angles ∠A and ∠B. Since ∠A and ∠B are corresponding angles, they form a linear pair.
Applications of Linear Pairs

Linear pairs have numerous applications in various fields, including architecture, engineering, and design. Some of the key applications of linear pairs include:
- Building Design: Linear pairs are used in building design to create symmetrical and aesthetically pleasing structures.
- Bridge Construction: Linear pairs are used in bridge construction to ensure stability and balance.
- Graphic Design: Linear pairs are used in graphic design to create visually appealing compositions.
5. Angle-Angle Similarity Theorem
The Angle-Angle Similarity Theorem is a fundamental concept in geometry that relies on linear pairs. The theorem states that if two angles of one triangle are congruent to two angles of another triangle, then the two triangles are similar. Linear pairs play a crucial role in this theorem, as they help establish the congruence of angles between two triangles.
What is a linear pair of angles?
+A linear pair of angles is a set of two adjacent angles that share a common vertex and lie on the same line.
What are the different types of linear pairs?
+Linear pairs can be classified into vertical linear pairs, adjacent linear pairs, and alternate interior linear pairs.
What is the Angle-Angle Similarity Theorem?
+The Angle-Angle Similarity Theorem states that if two angles of one triangle are congruent to two angles of another triangle, then the two triangles are similar.
In conclusion, linear pairs play a vital role in forming supplementary angles, and understanding their properties and applications is essential for solving geometric problems. By exploring the different types of linear pairs and their characteristics, we can gain a deeper understanding of geometric concepts and their real-world applications. Whether you're a student, teacher, or simply a geometry enthusiast, this article has provided you with a comprehensive overview of linear pairs and their significance in the world of geometry.