Understanding and working with polynomials is a fundamental skill in algebra and mathematics. A polynomial is an expression consisting of variables and coefficients combined using only addition, subtraction, and multiplication. The standard form of a polynomial is a way of writing it that makes it easy to read and work with. In this article, we will explore the master standard polynomial form in 5 easy steps, helping you to better understand and work with polynomials.
Step 1: Understanding the Basics of Polynomials

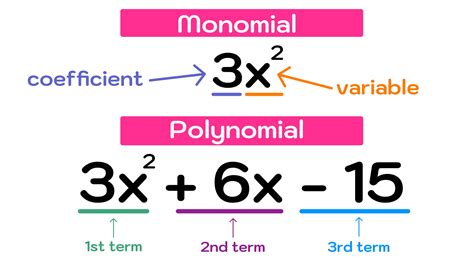
Before diving into the standard form, it's essential to understand the basics of polynomials. A polynomial is an expression that consists of variables and coefficients combined using only addition, subtraction, and multiplication. For example, 2x + 3y - 4 is a polynomial. Polynomials can have one or more terms, and each term is a combination of a coefficient and a variable(s) raised to a power.
Terminology
- Coefficient: A number that is multiplied by a variable(s).
- Variable: A letter or symbol that represents a value.
- Term: A single part of a polynomial, consisting of a coefficient and a variable(s) raised to a power.
- Degree: The highest power of the variable(s) in a polynomial.
Step 2: Identifying the Degree of the Polynomial

The degree of a polynomial is the highest power of the variable(s) in the polynomial. To identify the degree, look for the term with the highest power of the variable(s). For example, in the polynomial 2x^3 + 3x^2 - 4, the degree is 3, because the highest power of x is 3.
Why is the degree important?
- The degree of a polynomial determines the number of roots or solutions it has.
- The degree of a polynomial also determines the type of graph it will produce.
Step 3: Arranging Terms in Descending Order

To write a polynomial in standard form, arrange the terms in descending order of the power of the variable(s). This means that the term with the highest power of the variable(s) comes first, followed by the term with the next highest power, and so on. For example, the polynomial 2x^3 + 3x^2 - 4 can be written in standard form as 2x^3 + 3x^2 - 4.
Why is descending order important?
- Descending order makes it easy to identify the degree of the polynomial.
- Descending order also makes it easy to compare and contrast polynomials.
Step 4: Combining Like Terms

When writing a polynomial in standard form, combine like terms. Like terms are terms that have the same variable(s) raised to the same power. For example, 2x^3 + 3x^3 can be combined to form 5x^3.
Why is combining like terms important?
- Combining like terms simplifies the polynomial and makes it easier to read and work with.
- Combining like terms also makes it easier to identify patterns and relationships.
Step 5: Writing the Final Polynomial

Once you have identified the degree, arranged the terms in descending order, and combined like terms, you can write the final polynomial. The final polynomial should be in standard form, with the term with the highest power of the variable(s) first, followed by the term with the next highest power, and so on.
Example
- Original polynomial: 2x^3 + 3x^2 - 4
- Standard form: 2x^3 + 3x^2 - 4
By following these 5 easy steps, you can master the standard polynomial form and work with polynomials with confidence.
What is the standard form of a polynomial?
+The standard form of a polynomial is a way of writing it that makes it easy to read and work with. It involves arranging the terms in descending order of the power of the variable(s) and combining like terms.
Why is the degree of a polynomial important?
+The degree of a polynomial determines the number of roots or solutions it has. It also determines the type of graph it will produce.
How do I combine like terms in a polynomial?
+Like terms are terms that have the same variable(s) raised to the same power. To combine like terms, add or subtract the coefficients of the like terms.
We hope this article has helped you to understand and master the standard polynomial form. Do you have any questions or feedback? Please leave a comment below.