Standard Form Of A Line Equation Explained

Quick Links :

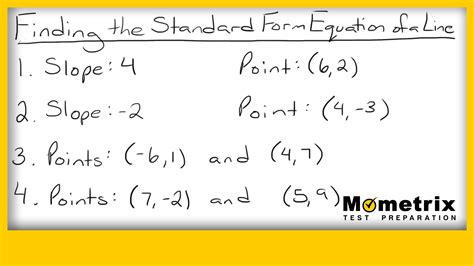
Lines are a fundamental concept in mathematics, particularly in algebra and geometry. They can be represented in various forms, but the standard form of a line equation is one of the most common and useful representations. In this article, we will delve into the world of line equations and explore the standard form, its significance, and how to work with it.

Lines are essentially sets of points that extend infinitely in two directions. They can be represented graphically on a coordinate plane, and their equations can be written in various forms, such as slope-intercept form, point-slope form, and standard form. Each form has its own advantages and disadvantages, but the standard form is particularly useful for solving systems of linear equations and for graphing lines.

What is the Standard Form of a Line Equation?

The standard form of a line equation is Ax + By = C, where A, B, and C are constants, and x and y are variables. In this form, A and B are coefficients of x and y, respectively, and C is the constant term. The standard form is also known as the general form of a line equation.

Significance of the Standard Form

The standard form of a line equation has several advantages over other forms. One of the main benefits is that it allows us to easily identify the x- and y-intercepts of the line. The x-intercept is the point where the line crosses the x-axis, and the y-intercept is the point where the line crosses the y-axis. By setting x = 0 and y = 0, respectively, we can find the intercepts.

Another advantage of the standard form is that it makes it easy to graph lines. By plotting the x- and y-intercepts, we can draw the line. Additionally, the standard form is useful for solving systems of linear equations. When we have two or more equations in standard form, we can use substitution or elimination methods to solve for the variables.

How to Convert Other Forms to Standard Form

To convert other forms of line equations to standard form, we need to manipulate the equation to get it in the form Ax + By = C. Here are some steps to follow:

• Slope-intercept form (y = mx + b): Multiply both sides by -1, then add b to both sides to get -mx + y = b.
• Point-slope form (y - y1 = m(x - x1)): Multiply both sides by -1, then add y1 to both sides to get -m(x - x1) + y = y1.

For example, let's convert the equation y = 2x + 3 to standard form. Multiply both sides by -1 to get -2x + y = -3, then add 3 to both sides to get -2x + y = 3.

Steps to Graph a Line in Standard Form

Graphing a line in standard form involves plotting the x- and y-intercepts, then drawing the line. Here are the steps to follow:

1. Set x = 0 and solve for y to find the y-intercept.
2. Set y = 0 and solve for x to find the x-intercept.
3. Plot the x- and y-intercepts on the coordinate plane.
4. Draw the line through the intercepts.

For example, let's graph the line 2x + 3y = 6. Set x = 0 and solve for y to get y = 2. Set y = 0 and solve for x to get x = 3. Plot the points (0, 2) and (3, 0), then draw the line through them.

Real-World Applications of Standard Form

The standard form of a line equation has numerous real-world applications in fields such as physics, engineering, economics, and computer science. Here are some examples:

• Physics: The standard form is used to describe the motion of objects, including the trajectory of projectiles and the path of celestial bodies.
• Engineering: The standard form is used to design and analyze systems, including electrical circuits and mechanical systems.
• Economics: The standard form is used to model economic systems, including supply and demand curves.
• Computer Science: The standard form is used in computer graphics and game development to create 2D and 3D models.

In conclusion, the standard form of a line equation is a powerful tool for solving systems of linear equations and graphing lines. Its advantages include ease of identifying x- and y-intercepts, graphing lines, and solving systems of equations. By understanding the standard form, we can unlock a wide range of applications in various fields.

Practice Exercises

Here are some practice exercises to help you master the standard form of a line equation:

• Convert the equation y = 3x - 2 to standard form.
• Graph the line 2x + 4y = 8.
• Solve the system of equations 2x + 3y = 7 and x - 2y = -3 using standard form.

We hope this article has helped you understand the standard form of a line equation and its significance. Remember to practice, practice, practice to become proficient in working with standard form.

We hope this article has helped you understand the standard form of a line equation. If you have any questions or comments, please feel free to share them below.