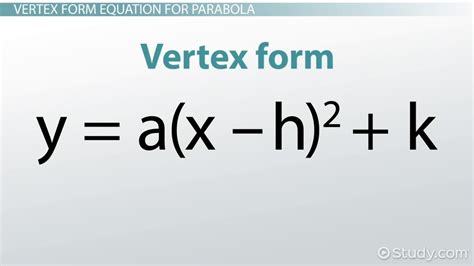
The parabola, a staple of mathematics and a crucial concept in various fields such as physics, engineering, and economics. When working with parabolas, it's essential to understand the standard form of a parabola, which is a fundamental concept in algebra and calculus. In this article, we'll delve into the world of parabolas, explore the standard form of a parabola, and provide you with a comprehensive guide on how to use a standard form of parabola calculator.
What is a Parabola?
A parabola is a type of quadratic curve that opens upwards or downwards. It's defined as the set of all points that are equidistant from a fixed point, known as the focus, and a fixed line, known as the directrix. Parabolas are commonly found in nature, architecture, and design, and they play a crucial role in various mathematical and scientific applications.

Standard Form of a Parabola
The standard form of a parabola is a mathematical representation of a parabola in a specific format. It's written as:
y = ax^2 + bx + c
where:
- y is the dependent variable (the output)
- x is the independent variable (the input)
- a is the coefficient of the squared term (affects the width and direction of the parabola)
- b is the coefficient of the linear term (affects the position and orientation of the parabola)
- c is the constant term (affects the position of the parabola)
The standard form of a parabola provides a concise and powerful way to describe and analyze parabolic curves.
Benefits of Standard Form
Using the standard form of a parabola offers several benefits, including:
- Simplified calculations: The standard form allows for easy calculations and manipulations of the parabola's equation.
- Clear visualization: The standard form provides a clear and concise way to visualize the parabola's shape and position.
- Efficient analysis: The standard form enables efficient analysis and comparison of different parabolas.
Standard Form of Parabola Calculator
A standard form of parabola calculator is a mathematical tool that helps you convert a parabola's equation into the standard form. These calculators are widely available online and can be used to:
- Convert a parabola's equation into the standard form
- Calculate the coefficients a, b, and c
- Visualize the parabola's shape and position

How to Use a Standard Form of Parabola Calculator
Using a standard form of parabola calculator is a straightforward process. Here are the steps:
- Enter the parabola's equation in the calculator's input field.
- Select the type of conversion you want to perform (e.g., convert to standard form).
- Click the calculate button.
- The calculator will display the parabola's equation in the standard form, along with the values of a, b, and c.
Example Problems
Here are some example problems to illustrate the use of a standard form of parabola calculator:
- Problem 1: Convert the equation y = 2x^2 + 3x - 4 into the standard form.
- Problem 2: Find the values of a, b, and c for the parabola y = x^2 - 2x + 1.

Conclusion
In conclusion, the standard form of a parabola is a powerful mathematical representation that provides a clear and concise way to describe and analyze parabolic curves. A standard form of parabola calculator is a valuable tool that helps you convert a parabola's equation into the standard form and calculate the coefficients a, b, and c.
We hope this article has provided you with a comprehensive guide on the standard form of a parabola and how to use a standard form of parabola calculator. Whether you're a student, teacher, or professional, we encourage you to try out the calculator and explore the fascinating world of parabolas.

Now it's your turn! Try out the standard form of parabola calculator and share your experiences with us. Do you have any questions or feedback? Leave a comment below and let's start a conversation.
What is the standard form of a parabola?
+The standard form of a parabola is y = ax^2 + bx + c, where a, b, and c are coefficients.
How do I use a standard form of parabola calculator?
+Enter the parabola's equation in the calculator's input field, select the type of conversion, and click the calculate button.
What are the benefits of using the standard form of a parabola?
+The standard form provides a clear and concise way to describe and analyze parabolic curves, simplifies calculations, and enables efficient analysis and comparison of different parabolas.