Ellipse Calculator In Standard Form Made Easy

Quick Links :

The ellipse is one of the most fundamental concepts in mathematics and physics, and it has numerous applications in various fields, including engineering, architecture, and astronomy. However, calculating the properties of an ellipse can be a daunting task, especially when it comes to standard form. In this article, we will delve into the world of ellipse calculators in standard form and explore how to make these calculations easy and efficient.

Understanding Ellipses in Standard Form

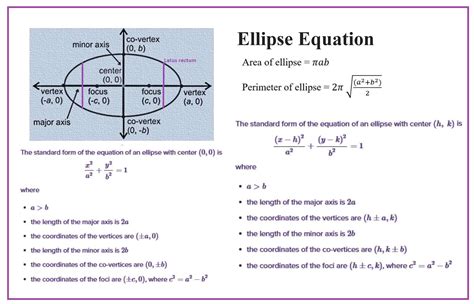
An ellipse is a closed curve on a plane surrounding two focal points such that the sum of the distances to the two focal points is constant. The standard form of an ellipse is given by the equation:

(x^2/a^2) + (y^2/b^2) = 1

where 'a' and 'b' are the lengths of the semi-major and semi-minor axes, respectively. The standard form of an ellipse provides a concise way to represent the ellipse's properties, including its shape, size, and orientation.

Benefits of Using an Ellipse Calculator in Standard Form

Using an ellipse calculator in standard form offers numerous benefits, including:

• Simplified calculations: Ellipse calculators can perform complex calculations quickly and accurately, saving time and reducing errors.
• Improved accuracy: Ellipse calculators can provide precise results, which is essential in applications where accuracy is critical, such as engineering and architecture.
• Increased efficiency: Ellipse calculators can perform multiple calculations simultaneously, making it an ideal tool for complex projects.

How to Use an Ellipse Calculator in Standard Form

Using an ellipse calculator in standard form is relatively straightforward. Here are the steps to follow:

1. Input the values of 'a' and 'b' into the calculator. These values represent the lengths of the semi-major and semi-minor axes, respectively.
2. Choose the desired calculation, such as calculating the area, perimeter, or focal distance of the ellipse.
3. Click the "Calculate" button to perform the calculation.
4. Review the results, which will be displayed in the calculator's output field.

Common Calculations for Ellipses in Standard Form

Here are some common calculations that can be performed using an ellipse calculator in standard form:

• Area: The area of an ellipse is given by the formula: A = πab
• Perimeter: The perimeter of an ellipse is given by the formula: P = 2π√((a^2 + b^2)/2)
• Focal distance: The focal distance of an ellipse is given by the formula: c = √(a^2 - b^2)

Practical Applications of Ellipse Calculators in Standard Form

Ellipse calculators in standard form have numerous practical applications in various fields, including:

• Engineering: Ellipse calculators are used in engineering to design and optimize systems, such as bridges, tunnels, and pipelines.
• Architecture: Ellipse calculators are used in architecture to design and optimize buildings, such as stadiums, arenas, and monuments.
• Astronomy: Ellipse calculators are used in astronomy to calculate the orbits of celestial bodies, such as planets and comets.

Real-World Examples of Ellipse Calculators in Standard Form

Here are some real-world examples of ellipse calculators in standard form:

• Designing a stadium: An architect uses an ellipse calculator to design a stadium with an elliptical shape. The calculator helps the architect to determine the optimal dimensions of the stadium, including the length and width of the ellipse.
• Calculating the orbit of a planet: An astronomer uses an ellipse calculator to calculate the orbit of a planet. The calculator helps the astronomer to determine the planet's distance from the sun and its velocity.

Conclusion: Making Ellipse Calculations Easy with Standard Form

In conclusion, using an ellipse calculator in standard form can make complex calculations easy and efficient. By understanding the benefits and applications of ellipse calculators, individuals can harness the power of these tools to simplify their work and improve accuracy. Whether you are an engineer, architect, or astronomer, an ellipse calculator in standard form is an essential tool to have in your toolkit.

We hope this article has provided you with a comprehensive understanding of ellipse calculators in standard form. If you have any questions or comments, please feel free to share them below.