5 Ways To Master Standard Form Complex Numbers

Quick Links :

Understanding and working with complex numbers is a fundamental aspect of various mathematical and scientific disciplines, including algebra, calculus, and electrical engineering. Among the ways to represent complex numbers, standard form is one of the most straightforward and widely used. In this article, we will delve into the world of complex numbers in standard form, exploring their definition, importance, and providing step-by-step guides on how to master their manipulation.

The importance of complex numbers cannot be overstated, especially in fields like physics and engineering, where they are used to describe phenomena that cannot be represented by real numbers alone. Complex numbers in standard form offer a convenient and algebraically rich way to perform calculations, solve equations, and analyze systems.

Understanding Complex Numbers in Standard Form

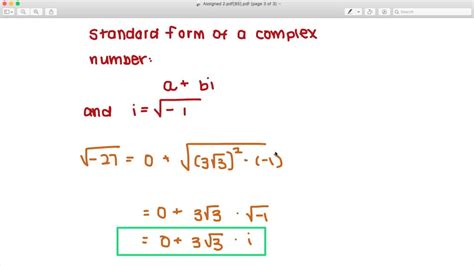
A complex number in standard form is represented as (a + bi), where (a) and (b) are real numbers and (i) is the imaginary unit with the property that (i^2 = -1). Here, (a) is the real part, and (bi) is the imaginary part. The standard form allows for easy addition, subtraction, multiplication, and division of complex numbers.

Importance of Complex Numbers in Standard Form

Complex numbers in standard form are crucial for several reasons:

• Simplification of Calculations: The standard form simplifies many algebraic operations, making it easier to perform calculations and solve equations involving complex numbers.
• Representation of Periodic Phenomena: Complex numbers are essential for representing periodic phenomena in physics and engineering, such as AC circuits and waveforms.
• Solutions to Algebraic Equations: Many algebraic equations have complex solutions, and understanding these solutions in standard form is vital for further analysis.

How to Add and Subtract Complex Numbers in Standard Form

Adding and subtracting complex numbers in standard form is straightforward, as you only need to add or subtract the real parts and the imaginary parts separately.

Steps for Addition:

1. Identify the real and imaginary parts of each complex number.
2. Add the real parts together.
3. Add the imaginary parts together.
4. Combine the results into a single complex number in standard form.

Steps for Subtraction:

1. Identify the real and imaginary parts of each complex number.
2. Subtract the real part of the second number from the real part of the first number.
3. Subtract the imaginary part of the second number from the imaginary part of the first number.
4. Combine the results into a single complex number in standard form.

How to Multiply Complex Numbers in Standard Form

Multiplying complex numbers in standard form involves using the distributive property and remembering that (i^2 = -1).

Steps for Multiplication:

1. Multiply each term of the first number by each term of the second number, using the distributive property.
2. Simplify the terms by remembering that (i^2 = -1).
3. Combine like terms and simplify further if possible.
4. Express the result in standard form.

How to Divide Complex Numbers in Standard Form

Dividing complex numbers in standard form requires multiplying both the numerator and denominator by the conjugate of the denominator.

Steps for Division:

1. Find the conjugate of the denominator by changing the sign of the imaginary part.
2. Multiply both the numerator and denominator by the conjugate of the denominator.
3. Simplify the numerator and denominator separately.
4. Express the result in standard form.

Practical Applications and Examples

Understanding and manipulating complex numbers in standard form has numerous practical applications in physics, engineering, and mathematics. For instance, in electrical engineering, complex numbers are used to analyze AC circuits and represent waveforms.

Example:

Consider an electrical circuit where the impedance is given by (Z = 3 + 4i) ohms. To find the current flowing through the circuit when a voltage of (V = 12 - 5i) volts is applied, you would use complex number division in standard form to solve for (I = \frac{V}{Z}).

Conclusion and Final Thoughts

Mastering complex numbers in standard form is a crucial skill for anyone working in or studying mathematical and scientific disciplines. By understanding the basics of complex numbers, how to add, subtract, multiply, and divide them, and seeing their practical applications, you can deepen your insight into the underlying principles of various phenomena. Whether you're an engineer analyzing circuits, a physicist modeling waveforms, or a mathematician solving equations, proficiency in complex numbers in standard form is an invaluable tool.

We hope this guide has provided a comprehensive introduction to working with complex numbers in standard form. We encourage you to practice the operations described here and explore the vast array of applications and uses of complex numbers in your field of interest.