5 Ways To Graph Parabolas In Standard Form

Quick Links :

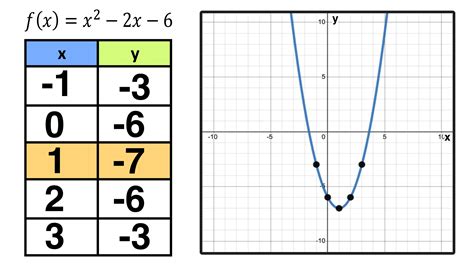
Understanding parabolas is a crucial aspect of algebra and geometry, as they are widely used to model various real-world phenomena, such as the trajectory of projectiles, the shape of satellite dishes, and the design of solar concentrators. A parabola in standard form is represented by the equation y = ax^2 + bx + c, where 'a', 'b', and 'c' are constants. In this article, we will explore five ways to graph parabolas in standard form, including identifying the vertex, axis of symmetry, and x-intercepts.

1. Identifying the Vertex

The vertex of a parabola is the lowest or highest point on the graph, depending on the direction of the parabola's opening. To identify the vertex, we need to determine the values of 'h' and 'k' in the vertex form of the parabola, which is given by y = a(x - h)^2 + k. We can do this by completing the square or using the formula h = -b/2a.

Once we have the values of 'h' and 'k', we can plot the vertex on the graph. The vertex is an essential point on the parabola, as it determines the direction and width of the parabola's opening.

Example:

Suppose we have a parabola in standard form: y = x^2 + 4x + 4. To identify the vertex, we need to complete the square:

y = x^2 + 4x + 4 y = (x^2 + 4x + 4) + 4 y = (x + 2)^2

Comparing this with the vertex form, we can see that h = -2 and k = 0. Therefore, the vertex is at the point (-2, 0).

2. Finding the Axis of Symmetry

The axis of symmetry is a vertical line that passes through the vertex of the parabola. To find the axis of symmetry, we can use the formula x = -b/2a.

Once we have the equation of the axis of symmetry, we can plot it on the graph. The axis of symmetry is essential, as it divides the parabola into two symmetric halves.

Example:

Suppose we have a parabola in standard form: y = x^2 + 4x + 4. To find the axis of symmetry, we can use the formula:

x = -b/2a x = -4/2(1) x = -2

Therefore, the axis of symmetry is the vertical line x = -2.

3. Determining the X-Intercepts

The x-intercepts are the points where the parabola intersects the x-axis. To determine the x-intercepts, we can set y = 0 and solve for x.

Example:

Suppose we have a parabola in standard form: y = x^2 + 4x + 4. To determine the x-intercepts, we can set y = 0 and solve for x:

x^2 + 4x + 4 = 0 (x + 2)^2 = 0 x + 2 = 0 x = -2

Therefore, the x-intercept is at the point (-2, 0).

4. Sketching the Parabola

Once we have identified the vertex, axis of symmetry, and x-intercepts, we can sketch the parabola. We can use these points to draw a smooth curve that opens upwards or downwards, depending on the value of 'a'.

Example:

Suppose we have a parabola in standard form: y = x^2 + 4x + 4. Using the points we have found, we can sketch the parabola:

Vertex: (-2, 0) Axis of symmetry: x = -2 X-intercept: (-2, 0)

Using these points, we can draw a smooth curve that opens upwards.

5. Using Graphing Software

Finally, we can use graphing software to graph parabolas in standard form. Graphing software can help us visualize the parabola and check our work.

Example:

Suppose we have a parabola in standard form: y = x^2 + 4x + 4. We can use graphing software to graph the parabola:

Using graphing software, we can see that the parabola opens upwards and has a vertex at (-2, 0).

In conclusion, graphing parabolas in standard form requires a combination of algebraic and geometric techniques. By identifying the vertex, axis of symmetry, and x-intercepts, we can sketch the parabola and visualize its shape. Additionally, we can use graphing software to check our work and explore different types of parabolas.

We hope this article has been helpful in understanding how to graph parabolas in standard form. If you have any questions or comments, please feel free to share them below.