Seventeen as a fraction in its simplest form can be expressed as 17/1, but let's explore a few more interesting aspects of fractions and how to simplify them.
The topic of fractions and simplifying them is a fundamental concept in mathematics, especially in the realm of algebra and arithmetic. Fractions represent a part of a whole, and understanding how to simplify them is crucial for various mathematical operations and real-world applications.
Understanding Fractions

Fractions are a way to express a part of a whole. They consist of two main parts: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, and the denominator tells us how many parts the whole is divided into.
For example, the fraction 3/4 represents three equal parts out of a total of four parts that make up the whole. Fractions can also be represented as decimals or percentages, but the fraction form is often more intuitive and easier to work with, especially when dealing with proportions and ratios.
Why Simplify Fractions?

Simplifying fractions is essential for several reasons:
- Clarity: Simplified fractions are easier to understand and work with. They provide a clearer representation of the proportion or ratio being described.
- Accuracy: When fractions are not in their simplest form, there's a higher chance of errors during mathematical operations. Simplifying fractions reduces this risk.
- Efficiency: Simplified fractions make calculations more efficient. They can speed up the process of solving equations and performing other mathematical tasks.
How to Simplify Fractions

Simplifying a fraction involves dividing both the numerator and the denominator by the greatest common divisor (GCD) until the GCD is 1. Here's a step-by-step guide:
- Identify the Fraction: Start with the fraction you want to simplify.
- Find the GCD: Calculate the greatest common divisor of the numerator and the denominator.
- Divide Both Numbers: Divide both the numerator and the denominator by the GCD.
- Repeat If Necessary: If the resulting fraction is not in its simplest form (i.e., the GCD is not 1), repeat steps 2 and 3 until it is.
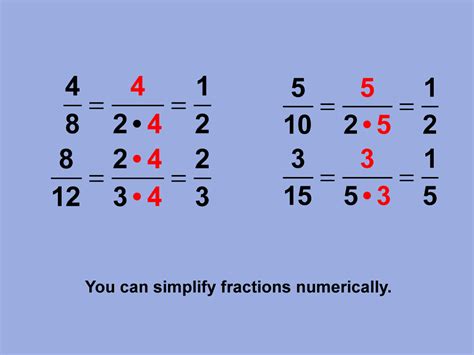
Example: Simplifying the Fraction 6/8

- The fraction is 6/8.
- The GCD of 6 and 8 is 2.
- Divide both 6 and 8 by 2, resulting in 3/4.
- The GCD of 3 and 4 is 1, meaning 3/4 is in its simplest form.
Conclusion and Further Learning

Fractions and their simplification are foundational concepts in mathematics, with applications ranging from simple arithmetic to advanced algebra and beyond. Understanding how to simplify fractions not only enhances mathematical proficiency but also aids in solving real-world problems involving proportions, ratios, and percentages.
For those looking to delve deeper, exploring topics such as equivalent fractions, mixed numbers, and improper fractions can further enhance understanding and application of fraction simplification.
What's Next?
Whether you're a student seeking to improve your grades, a teacher looking for resources, or simply someone curious about mathematics, there's always more to explore. Consider diving into:
- Mathematical Operations with Fractions: Learn how to add, subtract, multiply, and divide fractions, including how to find common denominators and deal with mixed numbers.
- Real-World Applications: Discover how fractions are used in everyday life, from cooking and finance to science and technology.
- Advanced Concepts: Explore more complex topics like fraction exponents, fraction inequalities, and solving equations involving fractions.
What is a fraction in simplest form?
+A fraction is in its simplest form when the greatest common divisor of the numerator and the denominator is 1.
Why is simplifying fractions important?
+Simplifying fractions is important for clarity, accuracy, and efficiency in mathematical operations.
How do I simplify a fraction?
+To simplify a fraction, divide both the numerator and the denominator by the greatest common divisor until the GCD is 1.