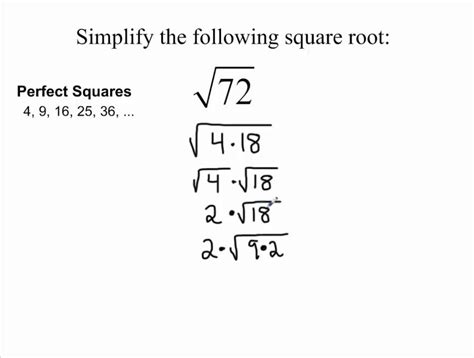
Simplifying the square root of 90 can seem like a daunting task, but it's actually quite straightforward. In this article, we'll break down the process into three easy steps, and by the end of it, you'll be a pro at simplifying square roots.
The square root of 90 is a number that, when multiplied by itself, gives us 90. To simplify it, we need to find the largest perfect square that divides 90. But before we dive into the steps, let's take a look at why simplifying square roots is important.
Why Simplify Square Roots?

Simplifying square roots is essential in various mathematical operations, such as solving equations, finding areas, and calculating distances. It helps us to express numbers in a more manageable form, making it easier to work with them. In real-life scenarios, simplifying square roots is crucial in fields like architecture, engineering, and physics, where accuracy is paramount.
Step 1: Find the Prime Factorization of 90

The first step in simplifying the square root of 90 is to find its prime factorization. We can do this by breaking down 90 into its prime factors:
90 = 2 × 3 × 3 × 5
This tells us that 90 is composed of the prime numbers 2, 3, and 5.
Why Prime Factorization is Important
Prime factorization is a crucial step in simplifying square roots because it helps us to identify the largest perfect square that divides the number. In this case, we can see that 3 × 3 = 9, which is a perfect square.
Step 2: Identify the Perfect Square

Now that we have the prime factorization of 90, we can identify the perfect square. As we mentioned earlier, 3 × 3 = 9 is a perfect square. We can rewrite 90 as:
90 = 9 × 10
This tells us that the largest perfect square that divides 90 is 9.
How to Identify Perfect Squares
To identify perfect squares, we need to look for pairs of prime factors that multiply to give a perfect square. In this case, we saw that 3 × 3 = 9, which is a perfect square.
Step 3: Simplify the Square Root

Now that we have identified the perfect square, we can simplify the square root of 90. We can rewrite the square root of 90 as:
√90 = √(9 × 10)
Using the property of square roots, we can simplify this as:
√90 = √9 × √10
√90 = 3√10
And that's it! We've simplified the square root of 90 in three easy steps.
Why Simplifying Square Roots is Important
Simplifying square roots is essential in various mathematical operations, such as solving equations, finding areas, and calculating distances. It helps us to express numbers in a more manageable form, making it easier to work with them.
What is the square root of 90?
+The square root of 90 is √90 = 3√10.
How do I simplify the square root of 90?
+To simplify the square root of 90, find the prime factorization of 90, identify the perfect square, and simplify the square root using the property of square roots.
Why is simplifying square roots important?
+Simplifying square roots is essential in various mathematical operations, such as solving equations, finding areas, and calculating distances. It helps us to express numbers in a more manageable form, making it easier to work with them.
We hope this article has helped you to simplify the square root of 90 in three easy steps. Remember to practice regularly to become proficient in simplifying square roots. If you have any questions or need further clarification, please don't hesitate to ask. Share this article with your friends and family to help them simplify square roots with ease.