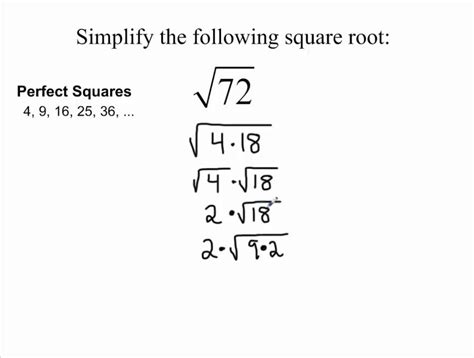
Simplifying square roots can seem like a daunting task, but with the right approach, it can be done in a few easy steps. In this article, we will walk you through the process of simplifying the square root of 8 in 5 easy steps.
What is a Square Root?

Before we dive into simplifying the square root of 8, let's take a quick look at what a square root is. A square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 16 is 4, because 4 multiplied by 4 equals 16.
Why Simplify Square Roots?

Simplifying square roots is an important skill in mathematics, as it helps to make calculations easier and more manageable. When working with square roots, it's often helpful to simplify them to their most basic form, which can make it easier to perform calculations and solve problems.
Step 1: Factor the Number

The first step in simplifying the square root of 8 is to factor the number. Factoring involves breaking down a number into its prime factors. In this case, the number 8 can be factored into 2 x 2 x 2, or 2^3.
What are Prime Factors?
Prime factors are the building blocks of numbers. They are the numbers that cannot be divided by any other number except for 1 and themselves. For example, the prime factors of 6 are 2 and 3, because 6 can be divided by 2 and 3, but not by any other number.Step 2: Look for Perfect Squares

The next step is to look for perfect squares in the factored form of the number. A perfect square is a number that can be expressed as the square of an integer. In this case, the factored form of 8 is 2 x 2 x 2, which can be rewritten as 2^2 x 2.
What is a Perfect Square?
A perfect square is a number that can be expressed as the square of an integer. For example, 16 is a perfect square because it can be expressed as 4^2.Step 3: Simplify the Square Root

Now that we have identified the perfect square in the factored form of 8, we can simplify the square root. The square root of 8 can be simplified as √(2^2 x 2), which can be rewritten as 2√2.
Step 4: Check for Further Simplification

The final step is to check if the simplified square root can be further simplified. In this case, the simplified square root of 8 is 2√2, which cannot be further simplified.
Step 5: Verify the Answer

The final step is to verify that the simplified square root is correct. We can do this by multiplying the simplified square root by itself to ensure that it equals the original number. In this case, 2√2 multiplied by 2√2 equals 8, which verifies that the simplified square root is correct.
What is the square root of 8?
+The square root of 8 is √8, which can be simplified to 2√2.
How do you simplify square roots?
+To simplify square roots, you need to factor the number, look for perfect squares, simplify the square root, check for further simplification, and verify the answer.
What is a perfect square?
+A perfect square is a number that can be expressed as the square of an integer. For example, 16 is a perfect square because it can be expressed as 4^2.
By following these 5 easy steps, you can simplify the square root of 8 and other numbers with ease. Remember to factor the number, look for perfect squares, simplify the square root, check for further simplification, and verify the answer. With practice, you'll become proficient in simplifying square roots and tackling more complex math problems.