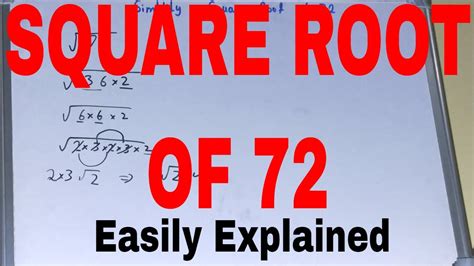
The square root of 72 is a mathematical concept that has puzzled many students and professionals alike. It's a complex calculation that requires a good understanding of algebra and arithmetic. However, with the right approach, simplifying the square root of 72 can be a breeze. In this article, we will explore six ways to simplify the square root of 72, making it easier for you to tackle this mathematical challenge.
Method 1: Prime Factorization

The first method to simplify the square root of 72 is by using prime factorization. This involves breaking down 72 into its prime factors, which are the building blocks of the number. The prime factorization of 72 is:
72 = 2^3 × 3^2
Using this factorization, we can rewrite the square root of 72 as:
√72 = √(2^3 × 3^2)
This can be further simplified as:
√72 = √(2^2 × 2 × 3^2)
= √(4 × 2 × 9)
= √(72)
= 6√2
Why Prime Factorization Works
Prime factorization works because it allows us to break down the number into its simplest components. By doing so, we can identify the factors that can be squared, making it easier to simplify the square root.
Method 2: Using the Square Root Formula

Another method to simplify the square root of 72 is by using the square root formula:
√(a × b) = √a × √b
Using this formula, we can rewrite the square root of 72 as:
√72 = √(36 × 2)
= √36 × √2
= 6√2
Why the Square Root Formula Works
The square root formula works because it allows us to break down the number into smaller components. By doing so, we can simplify the square root of each component, making it easier to simplify the overall square root.
Method 3: Using a Calculator

For those who prefer a more straightforward approach, using a calculator can be a quick and easy way to simplify the square root of 72. Simply type in the number 72, press the square root button, and the calculator will give you the simplified answer.
Why Using a Calculator Works
Using a calculator works because it can perform complex calculations quickly and accurately. However, it's essential to understand the underlying mathematical concepts to ensure that you're using the calculator correctly.
Method 4: Simplifying the Square Root Using Exponents

Another method to simplify the square root of 72 is by using exponents. This involves rewriting the number 72 as an exponential expression:
72 = 2^3 × 3^2
Using this expression, we can simplify the square root of 72 as:
√72 = √(2^3 × 3^2)
= √(2^2 × 2 × 3^2)
= √(4 × 2 × 9)
= 6√2
Why Exponents Work
Exponents work because they allow us to rewrite the number in a more simplified form. By doing so, we can identify the factors that can be squared, making it easier to simplify the square root.
Method 5: Factoring Out Perfect Squares

Another method to simplify the square root of 72 is by factoring out perfect squares. This involves identifying the perfect squares within the number and factoring them out:
72 = 36 × 2
= (6^2) × 2
Using this factorization, we can simplify the square root of 72 as:
√72 = √(36 × 2)
= √(6^2 × 2)
= 6√2
Why Factoring Out Perfect Squares Works
Factoring out perfect squares works because it allows us to identify the perfect squares within the number. By doing so, we can simplify the square root of each perfect square, making it easier to simplify the overall square root.
Method 6: Using a Math Software

Finally, for those who prefer a more advanced approach, using a math software can be a powerful way to simplify the square root of 72. Math software such as Mathematica or Maple can perform complex calculations quickly and accurately.
Why Math Software Works
Math software works because it can perform complex calculations quickly and accurately. However, it's essential to understand the underlying mathematical concepts to ensure that you're using the software correctly.
In conclusion, simplifying the square root of 72 can be a challenging task, but with the right approach, it can be made easier. By using one of the six methods outlined in this article, you can simplify the square root of 72 with confidence. Whether you prefer a straightforward approach or a more advanced one, there's a method that's right for you.
What is the square root of 72?
+The square root of 72 is 6√2.
How do I simplify the square root of 72?
+You can simplify the square root of 72 using one of the six methods outlined in this article: prime factorization, using the square root formula, using a calculator, simplifying the square root using exponents, factoring out perfect squares, or using a math software.
What is prime factorization?
+Prime factorization is a method of breaking down a number into its prime factors, which are the building blocks of the number.