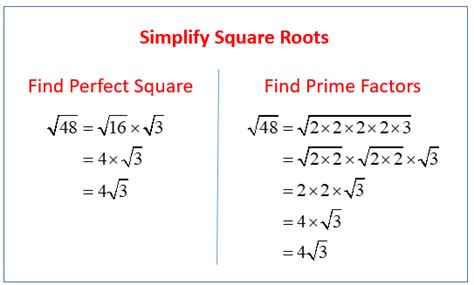
Simplifying square roots can seem daunting, but with a step-by-step approach, you can master the process. In this article, we'll break down the square root of 60 into simpler components using 5 easy steps.
Understanding Square Roots

Before we dive into simplifying the square root of 60, it's essential to understand what square roots represent. A square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 16 is 4, because 4 multiplied by 4 equals 16.
Step 1: Factorize the Number 60

To simplify the square root of 60, we need to factorize the number 60 into its prime factors. The prime factorization of 60 is:
60 = 2 × 2 × 3 × 5
Why is Factorization Important?
Factorization helps us identify the perfect squares within the number, making it easier to simplify the square root.
Step 2: Identify Perfect Squares

From the prime factorization of 60, we can identify the perfect squares:
2 × 2 = 4 (perfect square)
How Does This Help?
Recognizing the perfect squares within the factorization allows us to take the square root of those numbers, simplifying the expression.
Step 3: Take the Square Root of Perfect Squares

Now, we take the square root of the perfect square 4:
√4 = 2
This simplifies the expression to:
√60 = √(2 × 2 × 3 × 5) = 2√(3 × 5)
Step 4: Simplify the Radical Expression

We can further simplify the radical expression by combining the remaining factors:
2√(3 × 5) = 2√15
What's the Result?
The simplified square root of 60 is 2√15.
Step 5: Verify the Simplification

To verify our simplification, we can multiply 2√15 by itself:
(2√15) × (2√15) = 4 × 15 = 60
This confirms that our simplification is correct.
In Conclusion...
Simplifying the square root of 60 may seem challenging, but by following these 5 easy steps, you can break down complex radical expressions into more manageable components. Remember to factorize the number, identify perfect squares, take the square root of perfect squares, simplify the radical expression, and verify your simplification.
By mastering this process, you'll become more confident in tackling square root problems and improve your math skills.
What is the purpose of simplifying square roots?
+Simplifying square roots helps to reduce complex radical expressions into more manageable components, making it easier to work with and understand the underlying math concepts.
Can I simplify the square root of any number?
+Not all square roots can be simplified. However, by following the steps outlined in this article, you can simplify many square roots and develop a deeper understanding of radical expressions.
How do I verify my simplification?
+To verify your simplification, multiply the simplified radical expression by itself. If the result equals the original number, then your simplification is correct.