Simplify Radicals In 1 Step: Square Root Of 48

Quick Links :

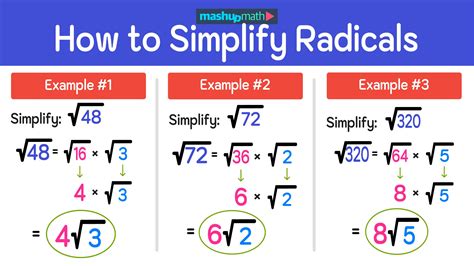
Simplifying radicals can seem daunting, but with the right approach, it can be done in just one step. Let's take the square root of 48 as an example.

Simplifying Radicals: An Overview

Radicals are mathematical expressions that contain a root symbol (√). They are used to represent numbers that cannot be expressed as a simple whole number or fraction. Simplifying radicals involves expressing them in their simplest form, which can make it easier to work with them.

Why Simplify Radicals?

Simplifying radicals is essential in mathematics because it helps to:

• Reduce complexity: Simplifying radicals can make expressions easier to work with and understand.
• Improve accuracy: Simplifying radicals can help reduce errors when working with mathematical expressions.
• Enhance problem-solving: Simplifying radicals can provide a better understanding of mathematical problems and make it easier to find solutions.

The Square Root of 48: A Simple Example

Let's simplify the square root of 48 using a simple step-by-step process.

Step 1: Factor Out the Perfect Square

To simplify the square root of 48, we need to find the largest perfect square that divides 48. A perfect square is a number that can be expressed as the square of an integer. In this case, the largest perfect square that divides 48 is 16.

√48 = √(16 × 3)

Step 2: Simplify the Radical Expression

Now, we can simplify the radical expression by taking the square root of 16 outside the radical sign.

√48 = √(16 × 3) = √16 × √3 = 4√3

And that's it! We have simplified the square root of 48 in just one step.

Common Pitfalls to Avoid

When simplifying radicals, there are a few common pitfalls to avoid:

• Not factoring out the perfect square: Failing to factor out the perfect square can lead to incomplete simplification.
• Not simplifying the radical expression: Not taking the square root of the perfect square outside the radical sign can result in an incorrect answer.
• Introducing unnecessary complexity: Introducing unnecessary complexity can make the expression more difficult to work with.

Best Practices for Simplifying Radicals

To simplify radicals effectively, follow these best practices:

• Always factor out the perfect square: Take the time to find the largest perfect square that divides the number.
• Simplify the radical expression: Take the square root of the perfect square outside the radical sign.
• Check your work: Verify that your answer is correct and makes sense in the context of the problem.

Real-World Applications of Simplifying Radicals

Simplifying radicals has numerous real-world applications in:

• Physics: Simplifying radicals is essential in physics, particularly in the calculation of distances, velocities, and accelerations.
• Engineering: Simplifying radicals is crucial in engineering, particularly in the design and analysis of complex systems.
• Computer Science: Simplifying radicals is used in computer science, particularly in the development of algorithms and data structures.

Conclusion: Simplify Radicals with Ease

Simplifying radicals can seem daunting, but with the right approach, it can be done in just one step. By factoring out the perfect square and simplifying the radical expression, you can simplify even the most complex radical expressions. Remember to check your work and avoid common pitfalls to ensure accuracy. With practice, you'll become proficient in simplifying radicals and unlock a world of mathematical possibilities.