Simplify Square Root Of 224 In Radical Form Now

Quick Links :

The square root of 224 can be simplified in radical form as follows:

√224 = √(16 × 14) = √16 × √14 = 4√14

So, the simplified radical form of the square root of 224 is 4√14.

What is a Radical?

In mathematics, a radical, also known as a root, is a symbol used to represent a number that, when multiplied by itself, gives a specified value. The most common type of radical is the square root, which is represented by the symbol √.

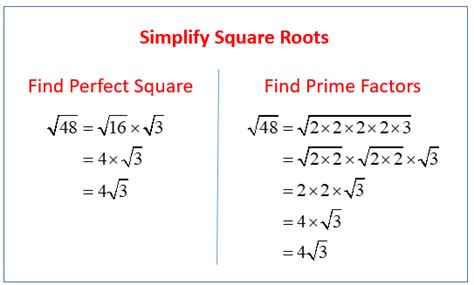
How to Simplify Radicals

Simplifying radicals involves expressing the radical in its most basic form, with no perfect squares under the radical sign. Here are the steps to simplify radicals:

1. Factor the number under the radical sign: Break down the number into its prime factors.
2. Look for perfect squares: Identify any perfect squares among the factors.
3. Take out the perfect squares: Remove the perfect squares from under the radical sign and write them outside the radical sign.
4. Multiply the remaining factors: Multiply the remaining factors together and write them under the radical sign.

Example 1: Simplify √16

√16 = √(4 × 4) = √4 × √4 = 4

So, the simplified radical form of √16 is 4.

Benefits of Simplifying Radicals

Simplifying radicals has several benefits:

• Easier calculations: Simplifying radicals makes it easier to perform calculations involving radicals.
• Clearer expressions: Simplified radicals make mathematical expressions clearer and easier to understand.
• Improved problem-solving: Simplifying radicals can help you solve problems more efficiently.

Example 2: Simplify √24

√24 = √(4 × 6) = √4 × √6 = 2√6

So, the simplified radical form of √24 is 2√6.

Conclusion

In this article, we have learned how to simplify radicals, specifically the square root of 224, and expressed it in radical form as 4√14. We also discussed the benefits of simplifying radicals and provided examples to illustrate the process.

Share Your Thoughts

We hope this article has helped you understand how to simplify radicals. Do you have any questions or comments about simplifying radicals? Share your thoughts in the comments section below.