5 Ways To Simplify Square Roots

Quick Links :

Square roots can be a daunting concept for many students, but with the right approach, they can be simplified and made more manageable. In this article, we will explore five ways to simplify square roots, making it easier for you to tackle math problems with confidence.

Mathematics is an essential subject that plays a crucial role in various aspects of our lives. From science and technology to finance and engineering, math is the foundation upon which many industries are built. However, for many students, math can be a source of anxiety and frustration. One of the most challenging concepts in math is square roots. Square roots can be a complex and abstract idea, making it difficult for students to grasp. Nevertheless, with the right strategies and techniques, square roots can be simplified, making it easier for students to understand and work with.

Square roots are an essential concept in mathematics, and their applications are numerous. In algebra, square roots are used to solve quadratic equations, while in geometry, they are used to calculate the length of sides of triangles. In real-life scenarios, square roots are used in physics, engineering, and computer science to model and analyze complex systems. Therefore, it is essential to have a solid understanding of square roots and how to simplify them.

Understanding Square Roots

Before we dive into the five ways to simplify square roots, it's essential to understand what square roots are and how they work. A square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 16 is 4, because 4 multiplied by 4 equals 16. Square roots can be denoted by the symbol √, and they can be either positive or negative.

Properties of Square Roots

Square roots have several properties that make them useful in mathematics. One of the most important properties of square roots is that they can be added and subtracted just like numbers. However, when multiplying and dividing square roots, the rules are slightly different. When multiplying square roots, we multiply the numbers inside the square root signs and then take the square root of the result. When dividing square roots, we divide the numbers inside the square root signs and then take the square root of the result.

Method 1: Simplifying Square Roots Using Perfect Squares

One of the simplest ways to simplify square roots is by using perfect squares. A perfect square is a number that can be expressed as the square of an integer. For example, 16 is a perfect square because it can be expressed as 4^2. When simplifying square roots using perfect squares, we look for perfect square factors of the number inside the square root sign.

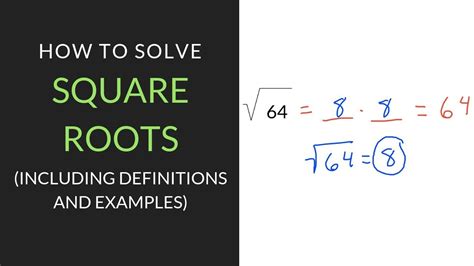
Example: Simplifying √16

Using the property of perfect squares, we can simplify √16 by finding the perfect square factors of 16. In this case, 16 can be expressed as 4^2, so we can simplify √16 as √(4^2) = 4. This method is useful when dealing with perfect squares, but it may not work for all numbers.

Method 2: Simplifying Square Roots Using Prime Factorization

Another way to simplify square roots is by using prime factorization. Prime factorization involves breaking down a number into its prime factors, which are the building blocks of numbers. When simplifying square roots using prime factorization, we look for pairs of identical prime factors.

Example: Simplifying √48

Using prime factorization, we can break down 48 into its prime factors: 48 = 2^4 × 3. We can then look for pairs of identical prime factors, in this case, 2^2 × 3. So, we can simplify √48 as √(2^2 × 3) = 2√3.

Method 3: Simplifying Square Roots Using Conjugate Pairs

Conjugate pairs are a useful tool for simplifying square roots. Conjugate pairs involve multiplying the numerator and denominator of a fraction by the conjugate of the denominator.

Example: Simplifying √(2 + √3)

Using conjugate pairs, we can simplify √(2 + √3) by multiplying the numerator and denominator by the conjugate of the denominator, which is (2 - √3). This results in √(2 + √3) = (2 + √3) / (2 - √3).

Method 4: Simplifying Square Roots Using Rational Exponents

Rational exponents are another way to simplify square roots. Rational exponents involve expressing a number as a fraction with a numerator and a denominator.

Example: Simplifying √(4/9)

Using rational exponents, we can simplify √(4/9) by expressing it as (4/9)^(1/2) = (2/3).

Method 5: Simplifying Square Roots Using Technology

Finally, technology can be a useful tool for simplifying square roots. Calculators and computer software can quickly and accurately calculate square roots, making it easier to simplify complex math problems.

Example: Simplifying √(256)

Using technology, we can simplify √(256) by using a calculator or computer software. The result is √(256) = 16.

In conclusion, simplifying square roots can be a challenging task, but with the right strategies and techniques, it can be made more manageable. By using perfect squares, prime factorization, conjugate pairs, rational exponents, and technology, you can simplify square roots with confidence.