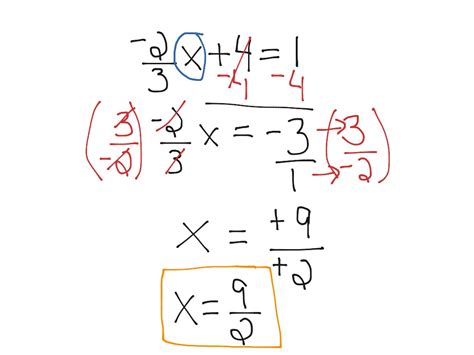Solving Multi-Step Fractional Equations With Ease

Quick Links :

Equations involving fractions can be intimidating, especially when they involve multiple steps. However, with the right approach and techniques, solving multi-step fractional equations can be made easier. In this article, we will explore the importance of mastering fractional equations, the benefits of breaking down complex equations, and provide a step-by-step guide on how to solve them.

Mastering fractional equations is crucial in various aspects of mathematics, science, and engineering. Fractions are used to represent proportional relationships between quantities, and equations involving fractions help us solve problems that involve scaling, ratios, and proportions. In real-world applications, fractional equations are used to model complex phenomena, such as population growth, chemical reactions, and electrical circuits. Therefore, it is essential to develop a strong foundation in solving fractional equations to excel in these fields.

Breaking down complex equations into manageable parts is a key strategy in solving multi-step fractional equations. By identifying the key components of the equation, such as the numerator, denominator, and any constants or coefficients, we can develop a plan to solve the equation step-by-step. This approach helps to reduce the complexity of the equation and makes it more manageable.

Understanding the Basics of Fractional Equations

Before diving into multi-step fractional equations, it is essential to understand the basics of fractional equations. A fractional equation is an equation that involves one or more fractions. The equation can be in the form of a simple fraction, such as 1/2, or a complex fraction, such as (x + 1)/(x - 1).

When solving fractional equations, we need to follow the order of operations, which is parentheses, exponents, multiplication and division (from left to right), and addition and subtraction (from left to right). We also need to be mindful of the rules of fractions, such as multiplying and dividing fractions by inverting and multiplying.

Types of Fractional Equations

There are several types of fractional equations, including:

• Simple fractional equations: These are equations that involve a single fraction, such as 1/2 = 3/4.
• Complex fractional equations: These are equations that involve multiple fractions, such as (x + 1)/(x - 1) = 2/3.
• Rational equations: These are equations that involve fractions with variables in the numerator and denominator, such as (x + 1)/(x - 1) = 2/x.

Step-by-Step Guide to Solving Multi-Step Fractional Equations

Solving multi-step fractional equations requires a systematic approach. Here is a step-by-step guide to help you solve these types of equations:

1. Read the equation carefully and identify the key components, such as the numerator, denominator, and any constants or coefficients.
2. Determine the type of equation you are dealing with, such as a simple fractional equation or a complex fractional equation.
3. Simplify the equation by canceling out any common factors in the numerator and denominator.
4. Use the rules of fractions to rewrite the equation in a more manageable form.
5. Isolate the variable by getting rid of any fractions or coefficients.
6. Solve for the variable using algebraic manipulations.
7. Check your solution by plugging it back into the original equation.

Example 1: Solving a Simple Fractional Equation

Suppose we have the equation 1/2 = 3/4. To solve this equation, we can follow the steps outlined above:

1. Read the equation carefully and identify the key components.
2. Determine the type of equation (simple fractional equation).
3. Simplify the equation by canceling out any common factors.
4. Use the rules of fractions to rewrite the equation in a more manageable form.
5. Isolate the variable (in this case, there is no variable).
6. Solve for the variable (not applicable).
7. Check the solution by plugging it back into the original equation.

By following these steps, we can see that the equation 1/2 = 3/4 is true, and there is no variable to solve for.

Example 2: Solving a Complex Fractional Equation

Suppose we have the equation (x + 1)/(x - 1) = 2/3. To solve this equation, we can follow the steps outlined above:

1. Read the equation carefully and identify the key components.
2. Determine the type of equation (complex fractional equation).
3. Simplify the equation by canceling out any common factors.
4. Use the rules of fractions to rewrite the equation in a more manageable form.
5. Isolate the variable by getting rid of any fractions or coefficients.
6. Solve for the variable using algebraic manipulations.
7. Check the solution by plugging it back into the original equation.

By following these steps, we can solve for the variable x and find the solution to the equation.

Common Challenges and Mistakes

When solving multi-step fractional equations, there are several common challenges and mistakes to watch out for:

• Canceling out common factors incorrectly
• Failing to simplify the equation
• Using the wrong rules of fractions
• Not isolating the variable correctly
• Making algebraic mistakes

To avoid these mistakes, it is essential to take your time, read the equation carefully, and follow the steps outlined above.

Conclusion and Final Thoughts

Solving multi-step fractional equations requires a systematic approach and a strong foundation in fractional equations. By breaking down complex equations into manageable parts, identifying the key components, and following the rules of fractions, we can solve these types of equations with ease. Remember to take your time, simplify the equation, and check your solution to avoid common mistakes. With practice and patience, you can master the art of solving multi-step fractional equations.

We hope this article has been helpful in providing a step-by-step guide to solving multi-step fractional equations. If you have any questions or comments, please feel free to share them below.