Solve Radicals In Seconds: 5 Easy Steps

Quick Links :

Mastering radicals is a fundamental skill for anyone looking to improve their math skills, and it's essential for success in various fields, including science, engineering, and finance. However, many people find radicals intimidating, and solving them can seem like a daunting task. But fear not! With the right approach, you can solve radicals in seconds. In this article, we'll break down the process into 5 easy steps, and by the end of it, you'll be a pro at solving radicals.

Radicals are an essential part of mathematics, and they play a critical role in various mathematical operations, including algebra, geometry, and calculus. However, radicals can be confusing, especially when it comes to simplifying them. But with the right techniques, you can simplify radicals with ease and solve them in seconds.

Step 1: Identify the Type of Radical

The first step in solving radicals is to identify the type of radical you're dealing with. There are two main types of radicals: square roots and nth roots. Square roots are the most common type of radical, and they're denoted by the symbol √. Nth roots, on the other hand, are denoted by the symbol ∛ or ∜, and they represent the nth root of a number.

Examples of Radicals

• √x (square root of x)
• ∛x (cube root of x)
• ∜x (fourth root of x)

Step 2: Simplify the Radical

Once you've identified the type of radical, the next step is to simplify it. Simplifying radicals involves factoring out perfect squares or perfect cubes from the radicand (the number inside the radical). For example, √16 can be simplified as √(4 x 4) = 4.

Examples of Simplifying Radicals

• √16 = √(4 x 4) = 4
• ∛27 = ∛(3 x 3 x 3) = 3
• ∜64 = ∜(4 x 4 x 4 x 4) = 4

Step 3: Rationalize the Denominator

When working with radicals, you'll often encounter fractions with radical denominators. To simplify these fractions, you need to rationalize the denominator by multiplying the numerator and denominator by a conjugate radical.

Examples of Rationalizing the Denominator

• 1/√2 = (1 x √2)/(√2 x √2) = √2/2
• 1/∛3 = (1 x ∛3)/(∛3 x ∛3) = ∛3/3

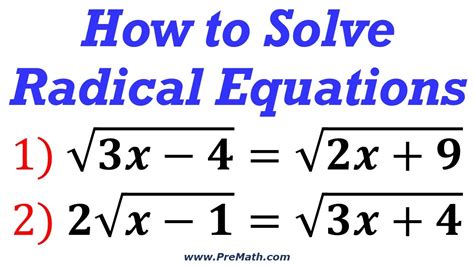
Step 4: Solve the Radical Equation

Now that you've simplified the radical and rationalized the denominator, the next step is to solve the radical equation. Radical equations involve solving for the variable inside the radical. For example, √x = 4 can be solved by squaring both sides of the equation: x = 16.

Examples of Solving Radical Equations

• √x = 4 => x = 16
• ∛x = 2 => x = 8
• ∜x = 3 => x = 81

Step 5: Check Your Solution

The final step is to check your solution by plugging it back into the original equation. This ensures that your solution is correct and that you haven't missed any steps.

Examples of Checking Your Solution

• √x = 4 => x = 16 => √16 = 4 (True)
• ∛x = 2 => x = 8 => ∛8 = 2 (True)
• ∜x = 3 => x = 81 => ∜81 = 3 (True)

By following these 5 easy steps, you can solve radicals in seconds and become a pro at working with radical equations. Remember to always simplify the radical, rationalize the denominator, and check your solution to ensure accuracy.

Now that you've mastered the art of solving radicals, it's time to put your skills to the test. Try solving some radical equations on your own, and don't forget to share your solutions with us in the comments below!