Simplify 28 To Simplest Radical Form In 2 Steps

Quick Links :

Simplifying Radicals in 2 Easy Steps

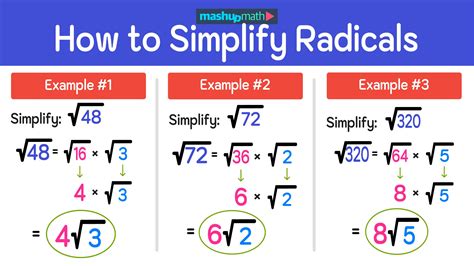
Simplifying radicals can seem like a daunting task, but with the right approach, it can be done in just 2 easy steps. In this article, we will explore the process of simplifying radicals, using the example of simplifying 28 to its simplest radical form.

Step 1: Find the Largest Perfect Square Factor

The first step in simplifying a radical is to find the largest perfect square factor of the number inside the radical. In the case of 28, we need to find the largest perfect square that divides 28. To do this, we can list the factors of 28 and identify the perfect squares:

• Factors of 28: 1, 2, 4, 7, 14, 28
• Perfect squares: 1, 4

As we can see, the largest perfect square factor of 28 is 4.

Step 2: Simplify the Radical

Now that we have identified the largest perfect square factor, we can simplify the radical. We can rewrite 28 as a product of 4 and another number:

28 = 4 × 7

Next, we can take the square root of 4, which is 2, outside of the radical:

√28 = √(4 × 7) = 2√7

And that's it! We have simplified 28 to its simplest radical form: 2√7.

Why Simplifying Radicals is Important

Simplifying radicals is an important skill in mathematics, particularly in algebra and geometry. It allows us to work with radicals in a more efficient and effective way. Here are some reasons why simplifying radicals is important:

• Easier calculations: Simplifying radicals makes calculations easier and faster. When working with radicals, we often need to perform calculations involving addition, subtraction, multiplication, and division. Simplifying radicals helps us to avoid complex calculations and reduces the risk of errors.
• Clearer understanding: Simplifying radicals helps us to understand the underlying structure of the radical. By breaking down a radical into its simplest form, we can gain insights into its properties and behavior.
• Better problem-solving: Simplifying radicals is an essential skill for problem-solving in mathematics. Many problems in algebra and geometry involve radicals, and being able to simplify them quickly and accurately is crucial for success.

Common Mistakes to Avoid When Simplifying Radicals

When simplifying radicals, there are several common mistakes to avoid:

• Not finding the largest perfect square factor: One of the most common mistakes is not finding the largest perfect square factor of the number inside the radical. This can lead to incorrect simplification and errors in calculations.
• Not taking the square root of the perfect square factor: Another common mistake is not taking the square root of the perfect square factor outside of the radical. This can result in an incorrect simplified form.
• Not simplifying the radical fully: Failing to simplify the radical fully can lead to incorrect answers and errors in calculations. Make sure to simplify the radical completely to avoid mistakes.

Conclusion: Mastering the Art of Simplifying Radicals

Simplifying radicals may seem like a challenging task, but with practice and the right approach, it can be done in just 2 easy steps. By following these steps and avoiding common mistakes, you can master the art of simplifying radicals and become more confident in your mathematical abilities. Remember to always find the largest perfect square factor and take the square root of that factor outside of the radical. With these skills, you'll be well on your way to simplifying radicals like a pro!