Simplifying Radicals: What Is The Simplest Radical Form Of 12

Quick Links :

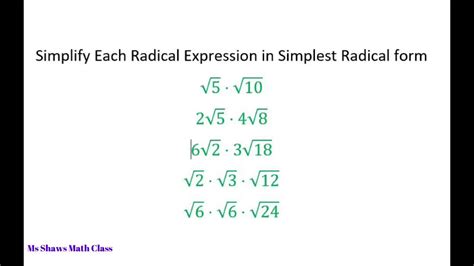
When it comes to simplifying radicals, many students and math enthusiasts often find themselves lost in a sea of complex calculations and confusing terminology. However, with a solid understanding of the basics and a step-by-step approach, simplifying radicals can become a breeze. In this article, we will explore the concept of simplifying radicals, specifically focusing on finding the simplest radical form of 12.

What are Radicals?

Before diving into the world of simplifying radicals, it's essential to understand what radicals are in the first place. In mathematics, a radical is a symbol used to represent the root of a number. The most common type of radical is the square root, denoted by the symbol √. Radicals can be used to represent various types of roots, such as cube roots, fourth roots, and so on.

Why Simplify Radicals?

Simplifying radicals is crucial in mathematics, particularly in algebra and geometry. When radicals are left in their complex form, they can be challenging to work with and may lead to errors in calculations. By simplifying radicals, we can make calculations more manageable, identify patterns, and solve problems more efficiently.

How to Simplify Radicals

Simplifying radicals involves factoring out perfect squares or perfect cubes from under the radical sign. Here are the general steps to follow:

1. Identify perfect squares or perfect cubes: Look for factors of the number under the radical sign that are perfect squares or perfect cubes.
2. Factor out the perfect square or perfect cube: Use the factored form to rewrite the radical expression.
3. Simplify the radical: If possible, simplify the radical further by factoring out any remaining perfect squares or perfect cubes.

Example: Simplifying the Radical of 12

Now that we have covered the basics of simplifying radicals, let's apply this knowledge to find the simplest radical form of 12.

√12 = √(4 × 3)

Since 4 is a perfect square, we can factor it out:

√12 = √4 × √3

Simplifying further, we get:

√12 = 2√3

As we can see, the simplest radical form of 12 is 2√3.

Benefits of Simplifying Radicals

Simplifying radicals offers numerous benefits, including:

• Improved accuracy: Simplifying radicals helps reduce errors in calculations and ensures that results are accurate.
• Enhanced problem-solving skills: By simplifying radicals, we can identify patterns and relationships between numbers, making it easier to solve problems.
• Increased efficiency: Simplifying radicals saves time and effort in calculations, allowing us to focus on more complex aspects of mathematics.

Real-World Applications of Simplifying Radicals

Simplifying radicals has numerous real-world applications, including:

• Physics and engineering: Simplifying radicals is crucial in calculating distances, velocities, and accelerations in physics and engineering.
• Computer science: Simplifying radicals is used in computer graphics, game development, and scientific simulations.
• Mathematical modeling: Simplifying radicals is essential in mathematical modeling, where complex systems are represented using mathematical equations.

Common Mistakes to Avoid When Simplifying Radicals

When simplifying radicals, it's essential to avoid common mistakes that can lead to errors in calculations. Some common mistakes include:

• Failing to identify perfect squares or perfect cubes: Not recognizing perfect squares or perfect cubes can lead to incomplete simplification.
• Incorrect factoring: Factoring incorrectly can result in incorrect simplification.
• Not simplifying further: Failing to simplify the radical further can lead to a more complex expression.

Conclusion

Simplifying radicals is an essential skill in mathematics, particularly in algebra and geometry. By understanding the basics of radicals, identifying perfect squares or perfect cubes, and following the steps to simplify radicals, we can make calculations more manageable and efficient. Remember to avoid common mistakes and apply the knowledge of simplifying radicals to real-world problems. With practice and patience, simplifying radicals can become a breeze.

We hope this article has helped you understand the concept of simplifying radicals and how to find the simplest radical form of 12. Share your thoughts and experiences in the comments section below!