Simplify Fractions In 3 Easy Steps

Quick Links :

Fractions are an essential part of mathematics, and understanding how to simplify them is a crucial skill for students and professionals alike. Whether you're working on a complex math problem or simply need to reduce a fraction to its simplest form, this article will guide you through the process in 3 easy steps.

Simplifying fractions is an important concept in mathematics, and it's used in a wide range of applications, from cooking and finance to science and engineering. By reducing fractions to their simplest form, you can make calculations easier, avoid confusion, and gain a deeper understanding of mathematical concepts.

In this article, we'll explore the importance of simplifying fractions, the benefits of reducing fractions to their simplest form, and provide a step-by-step guide on how to simplify fractions in 3 easy steps.

Why Simplify Fractions?

Simplifying fractions is essential in mathematics because it helps to:

• Reduce confusion: Simplifying fractions eliminates ambiguity and makes it easier to compare and calculate with fractions.
• Improve accuracy: Reducing fractions to their simplest form ensures that calculations are accurate and reliable.
• Enhance understanding: Simplifying fractions helps to reveal underlying mathematical concepts and relationships.

Step 1: Identify the Greatest Common Divisor (GCD)

To simplify a fraction, you need to find the greatest common divisor (GCD) of the numerator and denominator. The GCD is the largest number that divides both numbers without leaving a remainder.

Here are some steps to find the GCD:

• List the factors of the numerator and denominator.
• Identify the common factors.
• Choose the largest common factor, which is the GCD.

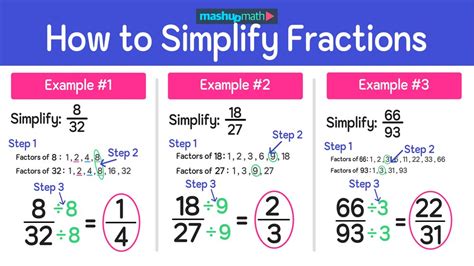
For example, if you want to simplify the fraction 12/16, you can find the GCD by listing the factors of 12 and 16:

Factors of 12: 1, 2, 3, 4, 6, 12 Factors of 16: 1, 2, 4, 8, 16

The common factors are 1, 2, and 4. The largest common factor is 4, which is the GCD.

Step 1.1: Divide the Numerator and Denominator by the GCD

Once you've found the GCD, divide the numerator and denominator by the GCD to reduce the fraction to its simplest form.

Using the example above, divide 12 and 16 by 4:

12 ÷ 4 = 3 16 ÷ 4 = 4

The simplified fraction is 3/4.

Step 2: Check for Any Remaining Common Factors

After dividing the numerator and denominator by the GCD, check if there are any remaining common factors. If there are, repeat the process of finding the GCD and dividing the numerator and denominator.

Using the example above, the simplified fraction is 3/4. However, there are no remaining common factors, so the fraction is already in its simplest form.

Step 2.1: Repeat the Process If Necessary

If there are remaining common factors, repeat the process of finding the GCD and dividing the numerator and denominator.

For example, if you want to simplify the fraction 18/24, you can find the GCD by listing the factors of 18 and 24:

Factors of 18: 1, 2, 3, 6, 9, 18 Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24

The common factors are 1, 2, 3, and 6. The largest common factor is 6, which is the GCD.

Divide 18 and 24 by 6:

18 ÷ 6 = 3 24 ÷ 6 = 4

The simplified fraction is 3/4. However, there are remaining common factors, so repeat the process.

Find the GCD of 3 and 4:

Factors of 3: 1, 3 Factors of 4: 1, 2, 4

The common factor is 1, which is the GCD.

Divide 3 and 4 by 1:

3 ÷ 1 = 3 4 ÷ 1 = 4

The simplified fraction is still 3/4.

Step 3: Verify the Simplified Fraction

Once you've simplified the fraction, verify that it's in its simplest form by checking if there are any remaining common factors.

Using the example above, the simplified fraction is 3/4. There are no remaining common factors, so the fraction is already in its simplest form.

In conclusion, simplifying fractions is an essential skill in mathematics that can help you reduce confusion, improve accuracy, and enhance understanding. By following these 3 easy steps, you can simplify fractions and make calculations easier.

We hope this article has helped you understand the importance of simplifying fractions and how to do it in 3 easy steps. If you have any questions or comments, please feel free to share them below.