5 Ways To Simplify Ratios Easily

Quick Links :

Ratios are a fundamental concept in mathematics, and simplifying them is a crucial skill that can help you solve problems more efficiently. Whether you're a student, a teacher, or a professional, being able to simplify ratios easily can save you time and effort in the long run. In this article, we'll explore five ways to simplify ratios easily, along with examples and explanations to help you master this skill.

Understanding Ratios

Before we dive into the methods for simplifying ratios, let's quickly review what a ratio is. A ratio is a way of comparing two or more numbers by division. It's often expressed as a fraction, with a colon (:) separating the numbers. For example, the ratio of apples to bananas in a fruit salad might be 3:4. This means that for every 3 apples, there are 4 bananas.

Why Simplify Ratios?

Simplifying ratios is important because it can help you:

• Compare different quantities more easily
• Identify equivalent ratios
• Solve problems more efficiently
• Make calculations simpler

Now, let's move on to the five ways to simplify ratios easily.

Method 1: Divide Both Numbers by the Greatest Common Divisor (GCD)

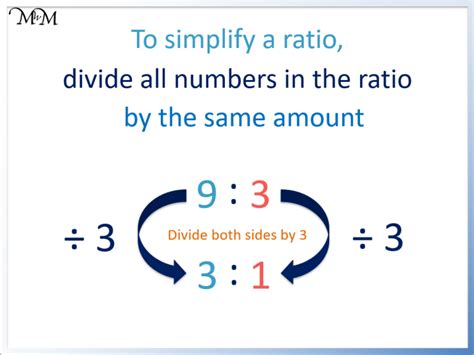
One of the simplest ways to simplify a ratio is to divide both numbers by their greatest common divisor (GCD). The GCD is the largest number that divides both numbers evenly.

For example, let's simplify the ratio 12:18.

1. Find the GCD of 12 and 18, which is 6.
2. Divide both numbers by 6: 12 ÷ 6 = 2, and 18 ÷ 6 = 3.
3. The simplified ratio is 2:3.

Method 2: Use the "Cancel Out" Method

The "cancel out" method involves canceling out common factors between the two numbers.

For example, let's simplify the ratio 9:12.

1. Look for common factors: both 9 and 12 have a factor of 3.
2. Cancel out the common factor: 9 ÷ 3 = 3, and 12 ÷ 3 = 4.
3. The simplified ratio is 3:4.

Method 3: Simplify Ratios with Prime Factors

Another way to simplify ratios is to use prime factors. This method involves breaking down each number into its prime factors and then simplifying.

For example, let's simplify the ratio 24:30.

1. Break down each number into prime factors: 24 = 2 × 2 × 2 × 3, and 30 = 2 × 3 × 5.
2. Cancel out common prime factors: both numbers have a factor of 2 × 3, so we can cancel those out.
3. The simplified ratio is 2:5.

Method 4: Use Equivalent Ratios

Equivalent ratios are ratios that have the same value, but are expressed differently. We can use equivalent ratios to simplify a given ratio.

For example, let's simplify the ratio 3:6.

1. Find an equivalent ratio: 3:6 is equivalent to 1:2, since both ratios have the same value.
2. Use the equivalent ratio: the simplified ratio is 1:2.

Method 5: Use Real-World Examples

Finally, we can use real-world examples to simplify ratios. This method involves using everyday situations to understand the ratio and simplify it.

For example, let's simplify the ratio of men to women in a company. Suppose the company has 250 men and 300 women.

1. Find the ratio: the ratio of men to women is 250:300.
2. Simplify the ratio using one of the methods above: let's use the "cancel out" method. Both numbers have a factor of 50, so we can cancel that out.
3. The simplified ratio is 5:6.

In conclusion, simplifying ratios is an essential skill that can help you solve problems more efficiently. By using one or more of the five methods outlined above, you can simplify ratios easily and accurately.

Whether you're a student, a teacher, or a professional, mastering the art of simplifying ratios can save you time and effort in the long run. So next time you come across a ratio, remember to simplify it using one of these methods, and watch how it makes your calculations simpler!

We hope this article has been helpful in explaining the five ways to simplify ratios easily. Do you have any questions or comments about simplifying ratios? Share them with us in the comments section below!