What Is A Ratio In Fraction Form

Quick Links :

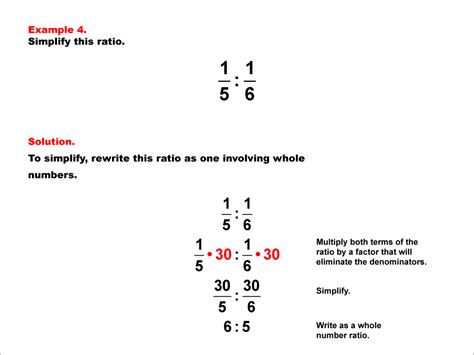
A ratio is a way of expressing the relationship between two quantities. It can be expressed in various forms, including fraction form, which is a widely used and intuitive way to represent ratios.

Understanding Ratios in Fraction Form

A ratio in fraction form is written as a/b, where a and b are the two quantities being compared. For example, if we have a ratio of 3:4, it can be expressed in fraction form as 3/4. In this example, a = 3 and b = 4.

Key Characteristics of Ratios in Fraction Form

1. Part-to-Part: A ratio in fraction form compares a part of one quantity to a part of another quantity.
2. Part-to-Whole: A ratio in fraction form can also compare a part of one quantity to the whole of another quantity.
3. Equivalent Ratios: Ratios in fraction form can be equivalent, even if they are not identical. For example, 1/2 and 2/4 are equivalent ratios.

How to Write a Ratio in Fraction Form

To write a ratio in fraction form, follow these steps:

1. Identify the two quantities being compared.
2. Determine the relationship between the two quantities.
3. Write the ratio as a/b, where a is the first quantity and b is the second quantity.

Examples of Writing Ratios in Fraction Form

1. Simple Ratio: A recipe calls for 2 cups of flour and 1 cup of sugar. The ratio of flour to sugar can be written as 2/1.
2. Complex Ratio: A car travels 250 miles in 5 hours. The ratio of miles to hours can be written as 250/5 or 50/1.

Advantages of Using Ratios in Fraction Form

Using ratios in fraction form has several advantages:

1. Simplifies Complex Relationships: Ratios in fraction form can simplify complex relationships between quantities.
2. Facilitates Comparisons: Ratios in fraction form make it easy to compare different quantities.
3. Enables Equivalent Ratios: Ratios in fraction form allow for equivalent ratios, which can be useful in various mathematical applications.

Real-World Applications of Ratios in Fraction Form

1. Cooking and Recipes: Ratios in fraction form are widely used in cooking and recipes to ensure the right proportions of ingredients.
2. Science and Engineering: Ratios in fraction form are used in science and engineering to express relationships between physical quantities, such as force and distance.
3. Finance and Economics: Ratios in fraction form are used in finance and economics to express relationships between financial quantities, such as investment returns and risk.

Common Mistakes When Working with Ratios in Fraction Form

When working with ratios in fraction form, common mistakes include:

1. Reversing the Order: Reversing the order of the quantities in a ratio can lead to incorrect results.
2. Ignoring Equivalent Ratios: Failing to recognize equivalent ratios can lead to incorrect conclusions.
3. Not Simplifying Fractions: Failing to simplify fractions can lead to complex and unnecessary calculations.

Best Practices for Working with Ratios in Fraction Form

1. Always Simplify Fractions: Simplify fractions to their lowest terms to avoid unnecessary calculations.
2. Check for Equivalent Ratios: Always check for equivalent ratios to ensure accurate conclusions.
3. Use Real-World Examples: Use real-world examples to illustrate the application of ratios in fraction form.

In conclusion, ratios in fraction form are a powerful tool for expressing relationships between quantities. By understanding the concept of ratios in fraction form, we can simplify complex relationships, facilitate comparisons, and enable equivalent ratios. Remember to always simplify fractions, check for equivalent ratios, and use real-world examples to illustrate the application of ratios in fraction form. Share your thoughts and examples of using ratios in fraction form in the comments below!