Understanding Polynomial Functions In Factored Form Easily

Quick Links :

Polynomial functions are a crucial part of algebra, and understanding them is essential for solving various mathematical problems. Among the different forms of polynomial functions, factored form is one of the most useful and widely used. In this article, we will delve into the world of polynomial functions in factored form, explore their benefits, and provide a step-by-step guide on how to work with them.

What are Polynomial Functions in Factored Form?

A polynomial function in factored form is a type of polynomial function that is written as a product of its factors. This form is particularly useful when we need to find the roots or zeros of the function, as it allows us to identify the factors that contribute to these values. In general, a polynomial function in factored form can be written as:

f(x) = a(x - r1)(x - r2)...(x - rn)

where a is a constant, and r1, r2,..., rn are the roots or zeros of the function.

Benefits of Polynomial Functions in Factored Form

Polynomial functions in factored form offer several benefits, including:

• Easy identification of roots or zeros: By factoring a polynomial function, we can easily identify the values of x that make the function equal to zero.
• Simplified algebraic manipulation: Factored form makes it easier to perform algebraic operations, such as multiplication and division, on polynomial functions.
• Improved problem-solving: Factored form can help us solve problems involving polynomial functions more efficiently, as it allows us to work with the individual factors rather than the entire function.

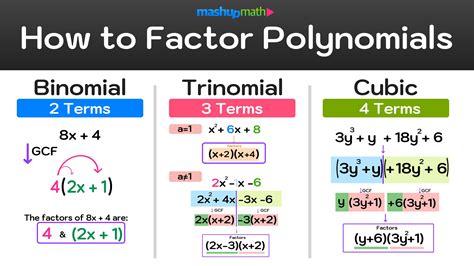
How to Factor Polynomial Functions

Factoring polynomial functions involves expressing them as a product of their factors. Here are the steps to factor a polynomial function:

1. Identify the degree of the polynomial: The degree of a polynomial is the highest power of x in the function.
2. Look for common factors: Check if there are any common factors among the terms of the polynomial.
3. Use factoring techniques: There are several factoring techniques, such as difference of squares, sum and difference, and factoring by grouping.
4. Check your work: Once you have factored the polynomial, check your work by multiplying the factors to ensure that you get the original polynomial.

Factoring Techniques

There are several factoring techniques that you can use to factor polynomial functions. Here are some of the most common techniques:

• Difference of squares: This technique involves factoring a polynomial that can be written as the difference of two squares.
• Sum and difference: This technique involves factoring a polynomial that can be written as the sum or difference of two cubes.
• Factoring by grouping: This technique involves factoring a polynomial by grouping terms and factoring out common factors.

Working with Polynomial Functions in Factored Form

Once you have factored a polynomial function, you can work with it in various ways. Here are some of the things you can do with polynomial functions in factored form:

• Find the roots or zeros: By setting each factor equal to zero, you can find the roots or zeros of the function.
• Perform algebraic operations: You can perform algebraic operations, such as multiplication and division, on polynomial functions in factored form.
• Solve problems: Factored form can help you solve problems involving polynomial functions more efficiently.

Examples of Polynomial Functions in Factored Form

Here are some examples of polynomial functions in factored form:

• f(x) = (x - 2)(x + 3)
• f(x) = (x^2 - 4)(x + 1)
• f(x) = (x - 1)(x - 2)(x - 3)

Conclusion

Polynomial functions in factored form are a powerful tool in algebra. By understanding how to factor polynomial functions, you can work with them more efficiently and solve problems more effectively. In this article, we have explored the benefits of polynomial functions in factored form, provided a step-by-step guide on how to factor them, and discussed how to work with them.