Point Slope Form Questions And Answers For Easy Understanding

Quick Links :

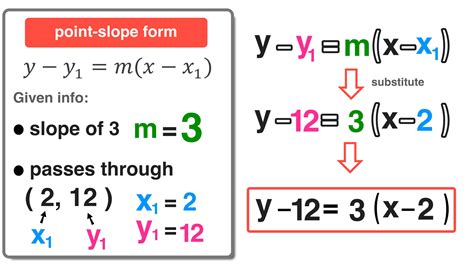
Understanding the point-slope form of a linear equation is crucial for algebra and other advanced math classes. It's a fundamental concept that helps you find the equation of a line given a point and the slope. In this article, we'll delve into the world of point-slope form, exploring its definition, benefits, and practical applications through questions and answers.

What is Point-Slope Form?

Point-slope form is a way to express the equation of a line. It's defined as y - y1 = m(x - x1), where m is the slope of the line, and (x1, y1) is a point on the line. This form is particularly useful when you know the slope and a point on the line.

Benefits of Using Point-Slope Form

The point-slope form offers several benefits over other forms of linear equations. For instance, it's easier to find the equation of a line when you're given the slope and a point. It's also useful when you need to find the equation of a line that's parallel or perpendicular to another line.

How to Use Point-Slope Form to Find the Equation of a Line

To use point-slope form to find the equation of a line, follow these steps:

• Identify the slope (m) and the point (x1, y1) on the line.
• Plug the values into the point-slope form equation: y - y1 = m(x - x1).
• Simplify the equation, if possible.

Example Problems

Let's look at a few example problems to illustrate how to use point-slope form.

• Find the equation of a line with a slope of 2 and passing through the point (3, 4).
• Solution: y - 4 = 2(x - 3). Simplifying the equation, we get y = 2x - 2.
• Find the equation of a line with a slope of -3 and passing through the point (-2, 5).
• Solution: y - 5 = -3(x + 2). Simplifying the equation, we get y = -3x - 1.

Practical Applications of Point-Slope Form

Point-slope form has numerous practical applications in various fields, including physics, engineering, economics, and computer science. For instance, it's used to model real-world phenomena, such as the motion of objects, population growth, and financial transactions.

Real-World Examples

• A car is traveling at a constant speed of 60 miles per hour. If it travels for 2 hours, how far will it go? We can use point-slope form to model this situation, where the slope represents the speed, and the point represents the initial position.
• A company's profit is increasing at a rate of $100 per day. If the initial profit is $500, what will be the profit after 5 days? We can use point-slope form to model this situation, where the slope represents the rate of increase, and the point represents the initial profit.

Common Mistakes to Avoid

When working with point-slope form, there are several common mistakes to avoid:

• Forgetting to include the negative sign when subtracting the y-coordinate of the point.
• Failing to simplify the equation, which can lead to incorrect solutions.
• Confusing the slope and the y-intercept.

Tips and Tricks

• Always label the point and the slope clearly when using point-slope form.
• Use online tools or graphing calculators to visualize the line and check your solutions.
• Practice, practice, practice! The more you practice using point-slope form, the more comfortable you'll become with it.

Conclusion and Final Thoughts

In conclusion, point-slope form is a powerful tool for finding the equation of a line. By understanding the benefits and practical applications of point-slope form, you'll become more confident in your ability to solve linear equations. Remember to avoid common mistakes, and don't be afraid to ask for help when needed.

We hope this article has helped you understand point-slope form better. If you have any questions or need further clarification, please leave a comment below. Share this article with your friends and classmates who may benefit from it.