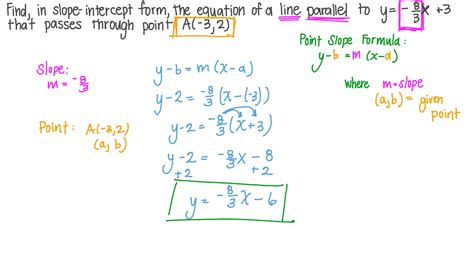
Point Slope Form For Parallel Lines Made Easy

Quick Links :

Point slope form is a powerful tool for finding the equation of a line, and when it comes to parallel lines, it can be a game-changer. Parallel lines are lines that never intersect, no matter how far they are extended. In this article, we'll explore how to use point slope form to find the equation of parallel lines with ease.

Understanding Parallel Lines

Parallel lines have the same slope, but they have different y-intercepts. This means that if you know the slope and a point on one of the lines, you can use point slope form to find the equation of the other line.

What is Point Slope Form?

Point slope form is a way of writing the equation of a line using the slope and a point on the line. The equation is written in the form:

y - y1 = m(x - x1)

where (x1, y1) is a point on the line and m is the slope.

Using Point Slope Form for Parallel Lines

To use point slope form for parallel lines, you need to know the slope and a point on one of the lines. Let's say you know the slope is 2 and a point on the line is (3, 4). You can use this information to find the equation of the line.

First, write the equation in point slope form:

y - 4 = 2(x - 3)

Next, simplify the equation by distributing the 2:

y - 4 = 2x - 6

Now, add 4 to both sides to get the equation in slope-intercept form:

y = 2x - 2

Benefits of Using Point Slope Form for Parallel Lines

Using point slope form for parallel lines has several benefits. For one, it makes it easy to find the equation of a line if you know the slope and a point on the line. It also makes it easy to find the equation of a parallel line if you know the slope and a point on the other line.

Additionally, point slope form can help you to identify the slope and y-intercept of a line, which can be useful in a variety of applications.

Examples of Point Slope Form for Parallel Lines

Here are a few examples of using point slope form for parallel lines:

Example 1:

Find the equation of a line that is parallel to the line y = 3x + 2 and passes through the point (2, 5).

Solution:

First, identify the slope of the line: m = 3

Next, write the equation in point slope form:

y - 5 = 3(x - 2)

Simplify the equation by distributing the 3:

y - 5 = 3x - 6

Now, add 5 to both sides to get the equation in slope-intercept form:

y = 3x - 1

Example 2:

Find the equation of a line that is parallel to the line y = 2x - 4 and passes through the point (1, 3).

Solution:

First, identify the slope of the line: m = 2

Next, write the equation in point slope form:

y - 3 = 2(x - 1)

Simplify the equation by distributing the 2:

y - 3 = 2x - 2

Now, add 3 to both sides to get the equation in slope-intercept form:

y = 2x + 1

Tips for Working with Point Slope Form for Parallel Lines

Here are a few tips to keep in mind when working with point slope form for parallel lines:

• Make sure you identify the slope correctly. If you know the slope of one line, you can use it to find the slope of a parallel line.
• Use the point slope form equation to find the equation of a line if you know the slope and a point on the line.
• Simplify the equation by distributing the slope and combining like terms.
• Use the equation in slope-intercept form to find the y-intercept and slope of the line.

Common Mistakes to Avoid

Here are a few common mistakes to avoid when working with point slope form for parallel lines:

• Forgetting to distribute the slope when simplifying the equation.
• Not combining like terms when simplifying the equation.
• Not identifying the slope correctly.
• Not using the correct point on the line.

By avoiding these common mistakes, you can ensure that you use point slope form correctly and effectively.

Conclusion

In conclusion, point slope form is a powerful tool for finding the equation of parallel lines. By understanding the benefits and using the equation correctly, you can make working with parallel lines a breeze. Remember to identify the slope correctly, use the point slope form equation, simplify the equation, and avoid common mistakes.

We hope this article has been helpful in making point slope form for parallel lines easy to understand. If you have any questions or comments, please feel free to share them below.